Students can Download Maths Chapter 10 Straight Lines Questions and Answers, Notes Pdf, 1st PUC Maths Question Bank with Answers helps you to revise the complete Karnataka State Board Syllabus and score more marks in your examinations.
Karnataka 1st PUC Maths Question Bank Chapter 10 Straight Lines
Question 1.
Define a point in a plane.
Answer:
A point in a plane is an ordered pair of real numbers.
If P = (x, y) then x and y are called the coordinates of P.
Important formulae
(i) If P = (x, y) then
x = the distance of P from y-axis = abscissa
y = the distance of P from x-axis = ordinate
(ii) Distance formulae
The distance between the points P(x1,y1) and Q (x2, y2) is given by
\( P Q=\sqrt{\left(x_{2}-x_{1}\right)^{2}+\left(y_{2}-y_{1}\right)^{2}}\)
The distance of the point (x, y) from the origin (0, 0) is \(\sqrt{x^{2}+y^{2}}\)
(iii) Section formulae
- The coordinates of the point which divides the line joining the points
(x1 y1) and (x2, y2) internally in the ratio m : n are
\(\left(\frac{m x_{2}+n x_{1}}{m+n}, \frac{m y_{2}+n y_{1}}{m+n}\right)\) - The coordinates of the point which divides the line joining the points externally in the ratio m : n are
\(\left(\frac{m x_{2}-n x_{1}}{m-n}, \frac{m y_{2}-n y_{1}}{m-n}\right)\)
In particular, if m = n, the coordinates of the mid-point of the line segment joining the points (x1 y1) and (x2, y2) are
\(\left(\frac{x_{2}+x_{1}}{2}, \frac{y_{2}+y_{1}}{2}\right)\)
(iv) Centroid of a triangle whose vertices are (x1 y1) (x2, y2) and (x3, y3) is
\(\left(\frac{x_{1}+x_{2}+x_{3}}{3}, \frac{y_{1}+y_{2}+y_{3}}{3}\right)\)
(v) Area of the triangle formed by the vertices (x1 y1) (x2, y2) and (x3, y3) is
\(\frac{1}{2}\left|x_{1}\left(y_{2}-y_{3}\right)+x_{2}\left(y_{3}-y_{1}\right)+x_{3}\left(y_{1}-y_{3}\right)\right|\)
If the third vertex is at the origin then area is \( \frac{1}{2}\left|x_{1} y_{2}-x_{2} y_{1}\right| \)
If the area of the triangle ABC is zero,then three points A, B and C lie in a line (i.e., they are collinear)
![]()
Question 2.
Define slope of a line.
Answer:
If a straight line makes an angle ‘θ’ with the positive direction of x-axis then ‘θ’ is called inclination of the line and ‘tan θ’ is called slope or gradient of the line. The slope of the line is denoted by ‘m’
∴ Slope = tan θ = m
Observations:
(i) 0 < θ < 180°
(ii) Slope of the line is a real number.
(iii) If θ = 90°, then the slope of the line is not defined (∵ tan 90° = ∞)
(iv) Slope of x-axis is zero
0 = 0 ⇒ tan θ = tan 0 = 0
(v)Slope of y-axis is not defined ∵ θ = 90°
⇒ tan θ = tan 90° is ∞
Question 3.
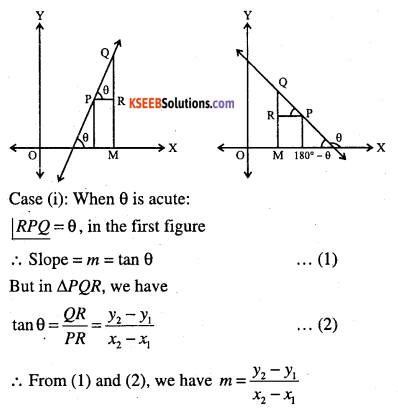
Prove that the slope of the line joining the points
\(\left(x_{1}, y_{1}\right) \text { and }\left(x_{2}, y_{2}\right) \text { is } m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}\)
Answer:
Let P((x1, y1) and Q(x2, y2) be two points on the line whose inclination be θ (θ may be acute or obtuse) Draw QM perpendicular to x-axis and PR perpendicular to QM.

Note: Three points A, B and C are collinear if and only if.
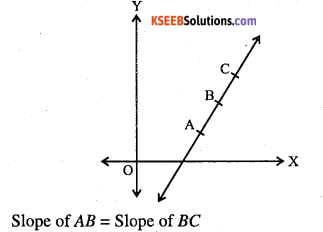
Question 4.
Show that two lines with slopes m1 and m2 are
(i) parallel iff m1 = m2
(ii) perpendicular iff m1 m2 = -1.
Answer:
(i) Let the lines l1 and l2 have slopes mi and m2 respectively and their respective inclinations be α and β.
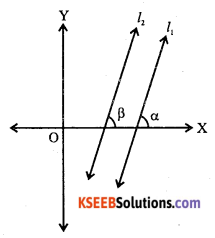
∴ tan α = m1 and tan β = m2
If l1 is parallel to l2 then α = β
∴ tan α = tan β
Conversely, if m1= m2, then
tan α = tan β ⇒ α = β lines are parallel
![]()
(ii) If l1 and l2 are perpendicular then P = 90° + α
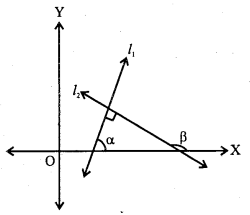
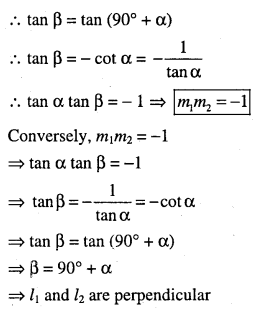
Question 5.
Derive the formula for finding the angle between the lines in terms of their slopes
Answer:
Let L1 and L2 be two non-vertical lines with slopes m1 and m2 respectively and a1 and a2 be the angles made by the lines L1 and L2 respectively.

∴ tan α1= m1 and tan a2 – m2
Let θ be the angle between the lines L1 and L2 and φ, adjacent angle to θ
From the figure,α2 = α1 + θ
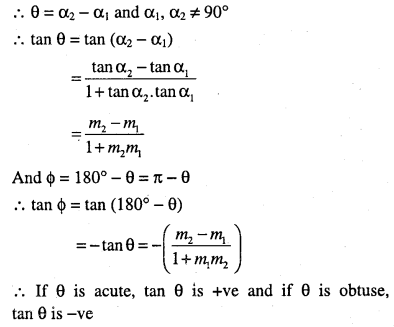
Note: The obtuse angle between the two given lines is π – θ.
Question 6.
Define intercepts made by the line with coordinate axes
Answer:
If a straight line cuts x-axis at and y-axis at B, then OA with proper sign is called x-intercept and OB with proper sign is called y-intercept made by the straight line.
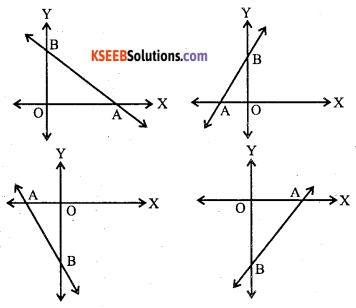
x-intercept is denoted by ‘a’ and y-intercept is denoted by ‘b’
Various forms (Different forms) of a line
Question 7.
Write the equations of x-axis and y-axis
Answer:
The x-axis is the locus of a point (x, y) which moves such that its y-coordinate is always zero.
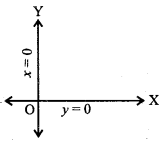
∴ Equation of x – axis is y = 0
Similarly, equation of y-axis is x = 0
![]()
Question 8.
Write the equations of horizontal line (parallel to x-axis) and vertical line (parallel toy-axis or perpendicular to x-axis)
Answer:
Let a horizontal line L is at a distance ‘a’ from the x-axis then the ordinate of every point lying on the line is either a or -a
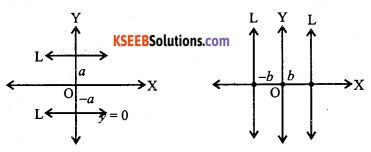
∴ Equation of the horizontal line is y = k
where k = a or – a
Similarly, equation of the vertical line is x = h where h = b or -b
Question 9.
Derive slope-intercept form of a line (with usual notations)
Or
Prove that the equation of a line with slope m and y-intercept y = mx + c.
Answer:
Let the line L with slope ‘m’ cuts the y-axis at a distance ‘c’ from the origin. Then y-intercept is ‘o’ and the line passes through A(0, c). Let P(x, y) be any point on the line. Then
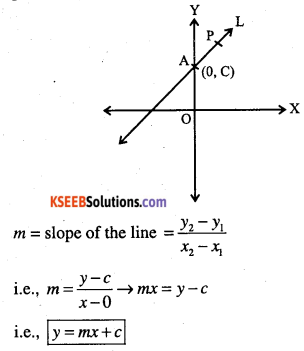
Note: This can be derived using slope-point form
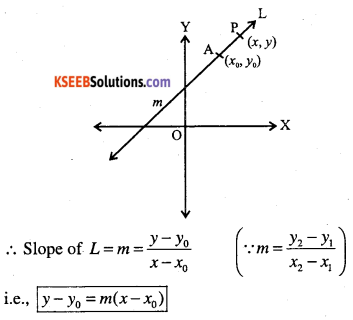
Question 10.
With usual, notations derive the equations of the line in the slope-point form as
y -y0 = m(x – x0)
OR
Prove that equation of a straight line with slope m and passing through the point
y -y0 = m(x – x0)
Answer:
Suppose that A(x0, yo) is a fixed point on a line L whose slope is m. Let P(x, y) be an arbitrary point on L.
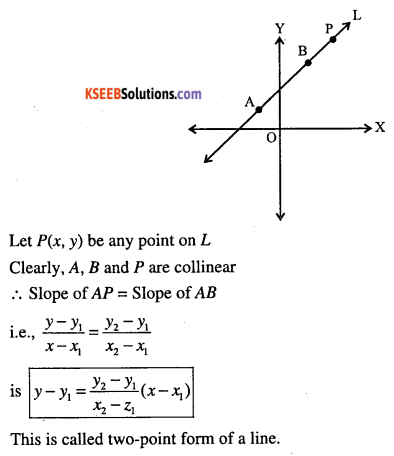
Question 11.
Prove that the equation of a line passing through the points (x1, y1) and (x2, y2) is
\(y-y_{1}=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}\left(x-x_{1}\right)\)
Answer:
Let the line L passes through the given point A(x1, y1)and B(x2, y2)
Question 12.
Prove that the equation of a line with x-intercept ‘a’ and y-intercept ‘b’ is \(\frac{x}{a}+\frac{y}{b}=1\) (Intercept form of a line)
Answer:
Because x-intercept is ‘a’
⇒ the line passes – through (a, 0)
y-intercept is b ⇒ the line passes through (0, b)

Question 13.
Prove that the equation of a line, ‘p’ as the length of the perpendicular drawn from origin and ‘w’ as the angle made by this perpendicular with positive directive of x-axis is x cos(w) + y sin(w) = p (Equation in the normal form)
Answer:
Let the line L meets x-axis at A and y-axis at B then required equation of the line is of the form .
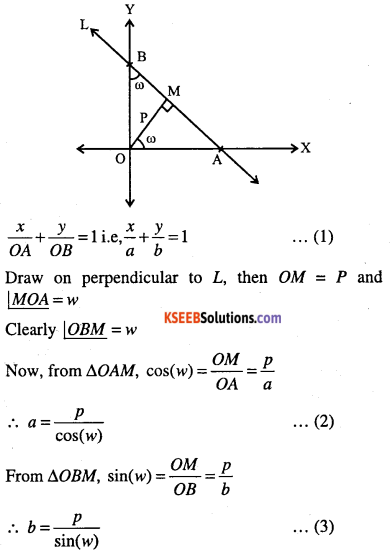
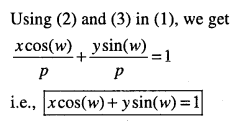
![]()
Question 14.
Write the general solution of a straight line and reduce it into slope-intercept form intercept and normal form. Find its slope and intercepts on the axes.
Answer:
Any equation of the form Ax + By + C = 0, where A and B are not zero simultaneously is a general solution of a line.


Remark:
If C = 0, then Ax + By = 0, which is a line passing through the origin and has zero intercepts on the axes.
iii. Normal form: Let x cos(w) + y sin(w) = p be the normal form of the line represented by the equation Ax +By+ C = 0 or Ax +By = -C
Thus both the equation are same
∴ Corresponding coefficients are in proportional
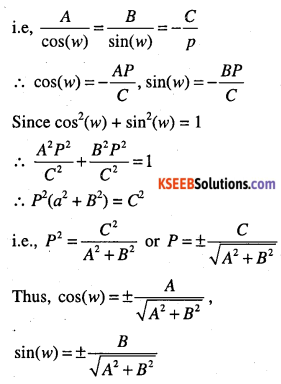
Question 15.
Define concurrent lines
Answer:
Three lines are said to be concurrent, if they pass through a common point.i.e., three lines are said to be concurrent if the point of intersection of two of them lies on the third line.
Question 16.
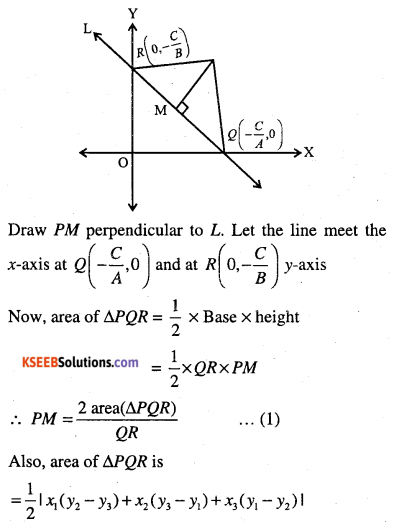
Derive the formula for the length of perpendicular drawn from any point (x1, y1) to the line Ax + By + C = 0
OR
Prove that the length of the perpendicular from any point
\(A x+B y+C=0 \text { is }\left|\frac{A x_{1}+B y_{1}+C}{\sqrt{A^{2}+B^{2}}}\right|\)
Answer:
Let L: Ax + By + C = 0 be a line, whose distance from the point P(x1, y1)is d.
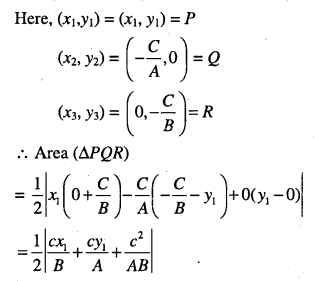

![]()
Question 17.
Show that the distance between two parallel lines y = mx + c1 and y = mx + c2 is
\(d=\left|\frac{c_{1}-c_{2}}{\sqrt{1+m^{2}}}\right|\)
Answer:
∴ Distance between l1 and l2 is equal to the length of the perpendicular from A to l2
∴ Distance between the lines l1 and l2 is

Question 18.
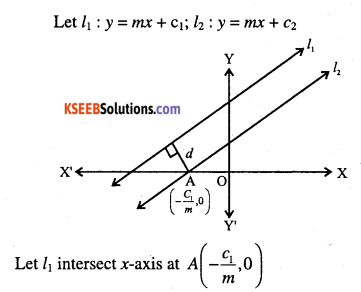
Show that the distance between two parallel lines ax + by + c1= 0 and ox + by + c2 = 0 is \(\left|\frac{c_{1}-c_{2}}{\sqrt{a^{2}+b^{2}}}\right|\)
Answer:
Let us assume that c1 and c2 are positive. Then the two lines lie on the same side of the origin
Let l1 : ax + by + c1 = 0 …(1)
And l2 : ax + by + c2 = 0 … (2)
Let ON and OM be the perpendicular distances from the origin to the lines l1 and l2 respectively.
Distance between parallel lines = MN = |ON – OM|
Now ON = length of perpendicular from O to l1
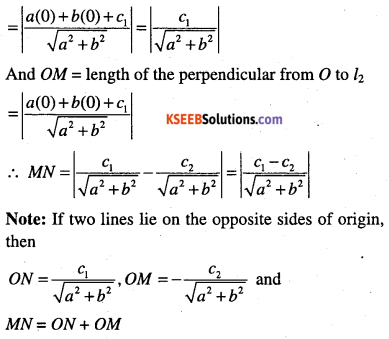
Question 19.
Show that equation of the line with slope ‘m’ makes x-intercept is y = m (x – d)
Answer:
Suppose a line with slope m cuts the x-axis at a distance d from the origin. Therefore point on the x-axis is (d, 0)

∴ By slope-point form, required equation of the line is of the form
y – y1= m (x – x1)
Here (x1, y1) = (d,0)
∴ (1) ⇒ y – 0 = m(x – d)
i.e., y = m(x-d)
![]()
Problems
Question 20.
Find the slope of the lines
(a) Passing through the points (3, -2) and (-1,4)
(b) Passing through the points (3, -2) and (7,-2)
(c) Passing through the points (3, -2) and (3,4)
Answer:
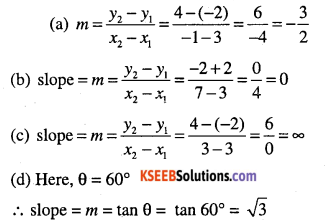
Question 21.
The base of an equilateral triangle with side 2a lies along y-axis such that the mid-point of the base is at the origin. Find the vertices of the triangle.
Answer:
As ‘O’ is the midpoint of the side BC of an equilateral triangle ABC, ‘A’ should lie on the x-axis
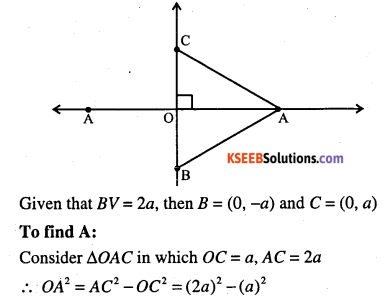
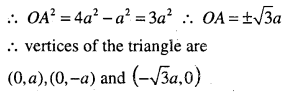
Question 22.
Find the distance between P(x1, y1) and Q(x2, y2) when
(i) PQ is parallel to y-axis
(ii) PQ is parallel to x-axis
Answer:
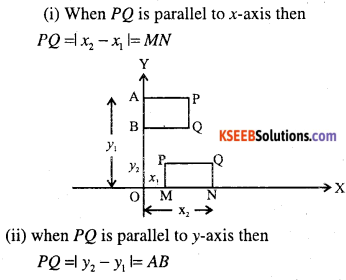
Question 23.
Find a point on the x-axis, which is equidistant from the points (7,6) and (3,4)
Answer:
Let A = (7, 6) and B = (3, 4) be the given points. P be a point on the x-axis The P is of the form (x, 0)
Given that P is equidistant from A and B i..e, AP = BP
Using distance formula, we have

Question 24.
Find the slope of a line, which passes through the origin and the mid-point of the line segment joining the point P(0, -4) and B (8,0)
Answer:
Given: 0(0,0), P(0, -4) and Q(8, 0)
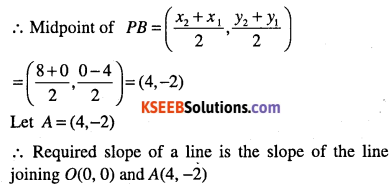
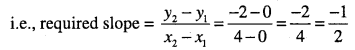
![]()
Question 25.
Without using Pythagoras theorem. Show that the points (4, 4), (3, 5) and (-1, -1) are the vertices of a right angled triangle
Answer:
Let A = (4, 4), B = (3, 5), C – (-1, -1) be the vertices of a ΔABC

Question 26.
Find the slope of the line, which makes an angle of 30° with the positive direction of y-axis measured anticlockwise.
Answer:
The line l makes an angle 30° with positive y-axis in the anticlockwise direction and it inclines 120° with the positive x-axis.
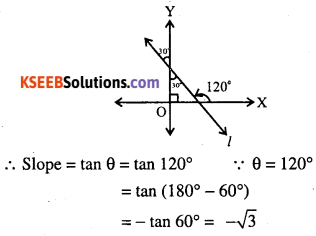
Question 27.
Find the value of x for which the points (x, -1), (2,1) and (4,5) are collinear
Answer:
Given points are collinear
⇒ Area of triangle formed by them is zero
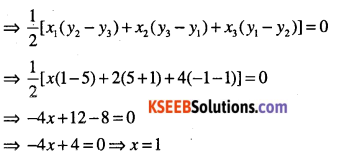
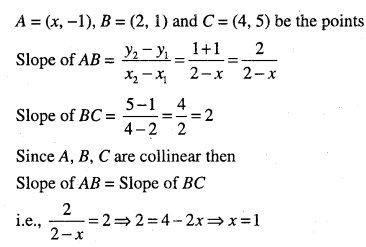
Question 28.
Without using distance formula, show that points (-2, -1), (4, 0) (3, 3) and (-3, 2) are the vertices of a parallelogram.
Answer:
Given points are A(-2, -1) B(4, 0), C(3, 3) and D(-3, 2)
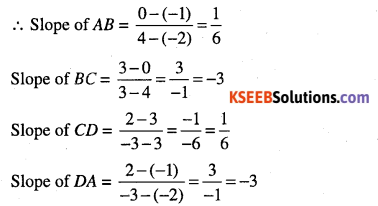
Clearly
Slope of AB = Slope of CD and Slope of BC = Slope of DA
∴ opposite sides of the quadrilateral ABCD are parallel
⇒ ABCD is a parallelogram
Question 29.
Find the angle between the x-axis and the line joining the points (3, -1) and (4, -2)
Answer:
The given points are A(3, -1) and B = (4, -2)
\(\text { Slope of } A B=\frac{-2-(-1)}{4-3}=\frac{-1}{1}=-1\)
Let θ be the angle made by the line AB with the positive x-axis then slope of
AB = tan θ … (2)
∴ From (1) and (2), we have
tan θ = -1 = – tan 45°
= tan (180°-45°)
= tan (135°)
= θ =135°
AB makes an angle of 135° with positive direction of x-axis
Question 30.
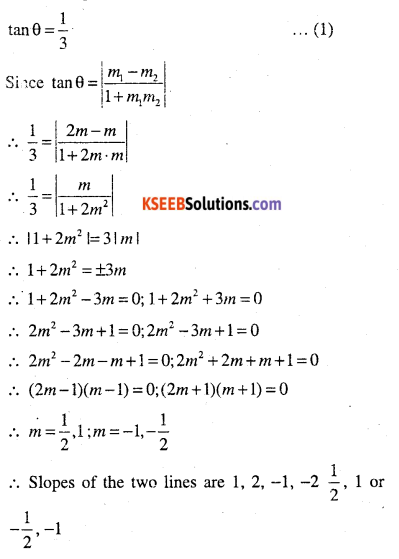
The slope of a line is double of the slope of the another line. If tangent of the angle between them is \(\frac{1}{3}\),find the slopes of the lines
Answer:
Let the slopes of the two lines be m and 2m and 0 be the angle between the lines. Then
Question 31.
If the angle between two lines is \(\frac{\pi}{4}\) and slope of one the lines \(\frac{1}{2}\)is find the slope of other line
Answer:
We have, the acute angle between two lines with slopes m1 and m2 is

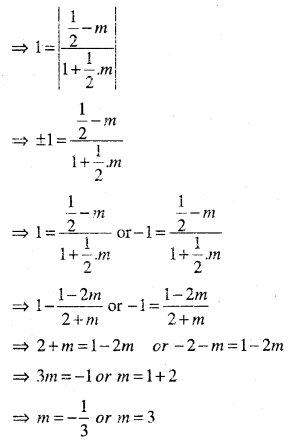
Question 32.
Line through the points (-2, 6) and (4, S) is perpendicular to the line through the points (8,12) and (x, 24). Find the value of x
Answer:
Slope of the line passing through the points (-2, 6) and (4, 8) is

![]()
Question 33.
A line passes through (x1, y1) and (h, k). If slope of the line is m, show that k-y1 =m(h-x1)
Answer:
Slope of the line passing through (x1 > y1) and (h, k) is
\(=\frac{k-y_{1}}{h-x_{1}}=m(\text { given })\)
k-y1=m(h-xl)
Question 34.
If the points (h, 0), (a, b) and (0, k) lie on a line, show that
\(\frac{a}{h}+\frac{b}{k}=1\)
Answer:
Let A(h, 0), B(a, b) and C(0, k) be the points lie on a line

Question 35.
Consider the following population and year graph find the slope of the line AB and using it, find what will be the population in the year 2010?
Answer:
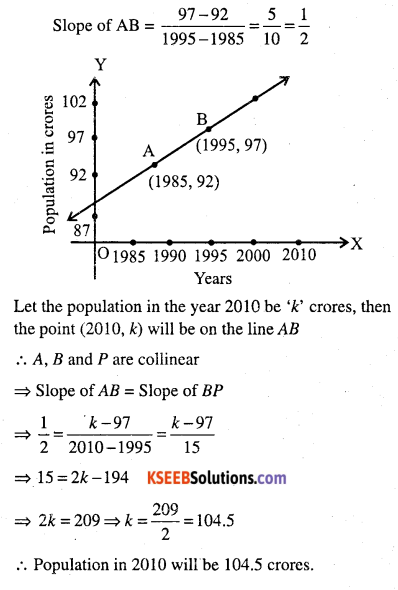
Problems on various forms of the equation of a line.
![]()
Question 36.
Find the equation of the line which satisfy the given conditions
(i) Write the equation for x and y axes
(ii) Passing through the point (-4, 3) with slope \(\frac{1}{2}\)
(iii) Passing through (0, 0) with slope m
(iv) Passing through \((2, \sqrt{3})\) and inclined with the x-axis at an angle of 15°.
(v) Intersecting the x-axis at a distance of 3 units to the left of origin with slope = -2
(vi) Intersecting the y-axis at a distance of 2 units above the origin and making an angle of 30° with positive direction of x-axis
(vii) Passing through the points (-1, 1) and (2,-4)
(viii) Perpendicular distance from the origin is 5 units and the angle made by the perpendicular with positive x-axis is 30°.
Answer:
(i) Equation of x-axis is y = 0
And equation of y-axis is x =0
(ii) Required equation is of the form

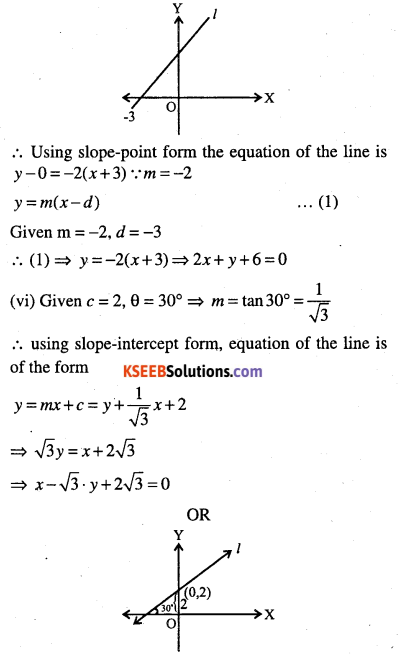
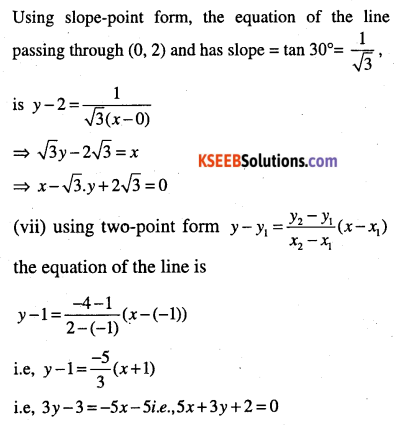
(viii) Given: Perpendicular from the orgin = p = 5 Angle made by the perpendicular and positive x – axis = w = 30º
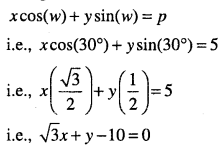
![]()
Question 37.
Find the equation of two line perpendicular distance from the origin is 4 units and the angle the normal makes with positive direction of x-axis is 15°
Answer:

Question 38.
Find the equation of the line, which makes -3 and 2 on the x and y-axes respectively.
Answer:
Given: x-intercept = a = -3
y – intercept = b = 2
∴ Using intercept form, required line is
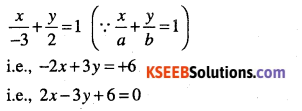
Question 39.
Write the equation of the lines for which \(\tan \theta=\frac{1}{2}, \theta\) is the inclination of the line and 2
(i) y-intercept is \(-\frac{3}{2}\)
(ii) x-intercept is 4.
Answer:
Using intercept form, required equations are of the form
y = mx+c and y = m(x-d)
\(\text { i.e., } y=\frac{1}{2} x-\frac{3}{2} \text { and } y=\frac{1}{2}(x-4)\)
![]()
Question 40.
Find the equation of the line passing through (-2,3) with slope -4.
Answer:
Given: (x1, y1) = (-2, 3), m = 4
Required equation is of the form
y – y1( = m(x-x1) (slope-point form)
i.e., y-3 = – 4(x + 2)
i.e., y-3 = – 4x – 8
i.e., 4x+y+5=0
Question 41.
Write the equation of the line through the points (1, -1) and (3,5)
Answer:
Given: (x1, y1)= (1, -1); (x2, y2) = (3, 5)
∴ Required equation is of the form

Question 42.
The vertices of ΔPQR are P(2,1) Q(-2, 3) and R(4, 5). Find the equation of the median triangle the vertex R
Answer:
Given P(2, 1), Q(-2, 3) and R(4, 5)
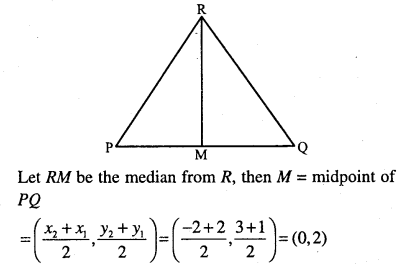
Equation of the median through R is the equation of the line through m(0, 2) and r(4, 5)
∴ By two-point form, equation of the line is
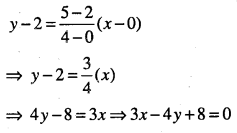
Question 43.
Find the equation of the line passing through (-3, 5) and perpendicular to the line through the points (2,5) and (-3,6)
Answer:
Given points are A(-3, 5), 5(2, 5) and C(-3, 6)
Now, Slope of BC =
\(B C=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{6-5}{-3-2}=-\frac{1}{5}\)
∴ Slope of any line perpendicular to BC = 5
(y condition for perpendicularity is m1 x m2 = -1)
The required equation of the line through A(-3, 5) with m- 5 is
y – 5 = 5(x + 3) (by slope-point form)
⇒ 5x-y + 20 = 0
Question 44.
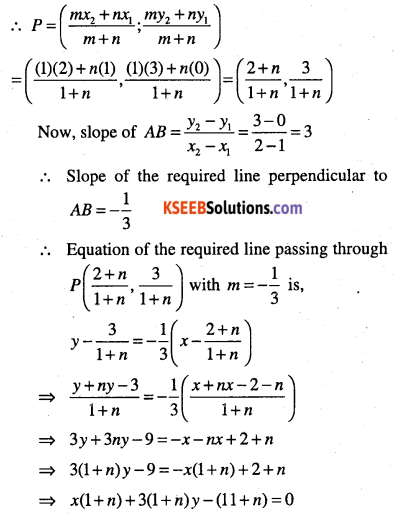
A line perpendicular to the line segment joining the points (1, 0) and (2, 3) divide it in the ratio 1: n. Find the equation of the line
Answer:
Let A(1, 0) and B (2, 3) be the given point
The required line divides the segment AB in the ratio 1 : n at P
Question 45.
Find the equation of the line that cuts off equal intercepts on the coordinate axes and passes through the point (2,3).
Answer:
Given : x-intercept = y-intercept
i.e., a = b, then the equation of the line is
\(\frac{x}{a}+\frac{y}{a}=1\)
⇒ x + y = a … (1)
Since (1) passes through the point (2, 3), then we have,
2 + 3 = a ⇒ a = 5
∴ From (1). required line is x + y = 5.
Question 46.
Find the equation of the line passing through (2, 2) and cutting off intercepts on the axes whose sum is 9.
Answer:
Let the intercepts on the axes be a and b
Given : a + b = 9 ⇒ b = 9-a
Intercepts of the line on the axes a, 9 – a
By intercept form, required line

Question 47.
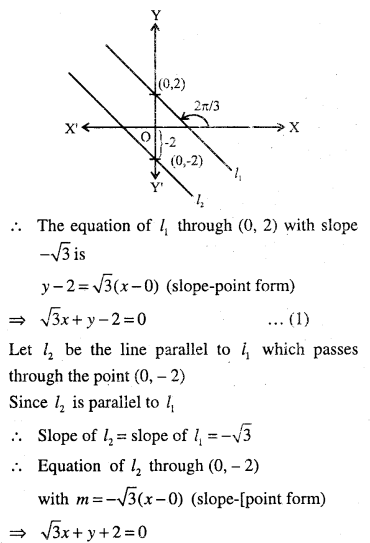
Find the equation of the line through the point (0, 2) making an angle \(\frac{2 \pi}{3} \)with positive x-axis. Also, find the equation of line parallel to it and crossing the y-axis at a distance of 2 units below the origin.
Answer:
Slope of the line

![]()
Question 48.
The perpendicular from the origin to a line meets it at the point (-2, 9), find the equation of the line.
Answer:
Let OM be the perpendicular drawn from 0 to 1

Question 49.
The length L cms of copper rod is a linear function of its Celsius temperature C, In an experiment, it L =942 when C = 20 and L = 125.134 when C = 110, express L in terms of C.
Answer:
Since L is a linear function of C then
L = mC + b ……………… (1)
(slope-intercept form) when C = 20, L = 124.942, then (1)
⇒ 124.924 = 20 m + b ……………… (2)
when C = 110, L = 125.134,then (1)
⇒ 125.134 = 110C + b ……………… (3)
From (2), 124,924 = 20 x 0.00213 + b
⇒ b = 124.942 – 0.00426 = 124.899
∴ (1) ⇒ L = 0.00213C + 124.899 is the required relation between L and C.
Question 50.
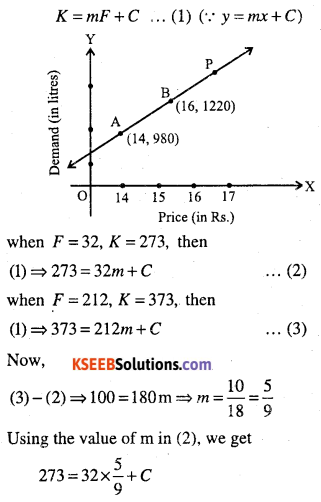
The Fahrenheit temperature F and absolute temperature K satisfy a linear equation. Give that K = 273 when F = 32 and that K = 373 when F = 212. Express K in terms of F and find the value of F, when K = 0.
Answer:
Take the equation in the form
Question 51.
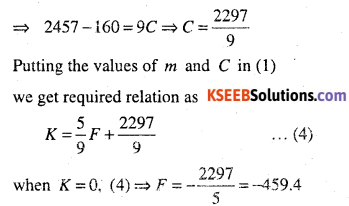
The owner of a milk store finds that he can sell 980 litres of milk each week at Rs. 14 litre and 1220 litres of milk each week at Rs. 16 litre. Assuming a linear relationship between selling price and demand how many litres could he sell weekly at Rs. 17 litre?
Answer:
Let the owner sell L litre of milk at Rs. p/ litre, then linear relationship between L and p be

OR
Let A=(14,980),B = (16,12200 then slope of
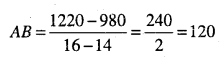
Let owner can sell L litres of milk at Rs. 17 per litre. Then the point P(17, L) will lie on the AB.
⇒ A, B and P are collinear
⇒ Sloe of AB = slope of BP
⇒ \(120=\frac{L-1220}{17-16}=L-1220\)
⇒ L = 120 + 1220 = 1340 litres
∴ The milk owner can sell 1340 litres of milk at Rs. 17 per litre.
Remark: This can be solve, using two-point form also.
![]()
Question 52.
P(a,b) is the midpoint of a line segment between axes. Show that equation of the line is
\(\frac{x}{a}+\frac{y}{b}=2\)
Answer:
Let the line meets the x-axis at A(h, 0) and y-axis at B(0, k), then the equation of the line segment of the line segment AB in intercept form is

Question 53.
Point R(h, k) divides a line segment between axes in the ratio 1 : 2. Find equation of the line.
Answer:
Let the line meets the x-axis at A and y-axis at B. Then
A = (a, 0) and B = (0, b)
Given : R divides AB in the ratio 1 : 2 then by section formula,

Question 54.
By using the concept of equation of a line, prove that the three points (3,0), (- 2, – 2) and (8,2) are collinear.
Answer:
Let A(3, 0), B(- 2, 2) and C(8, 2) be the given points
Using two-point form, we can find the equation of AB as

If C(8, 2) lies on the line (1) then we can conclude A, B and C are collinear.
Now putting x = 8 and y =2 in (1), we get
5(2) = 2(8) – 6
10 = 16 – 6= 10
10 – 10=0
= Clieson(1)
= A, B and C are collinear
![]()
Question 55.
Reduce the equations into slope-intercept form and And their sloes and y-intercept.
(i) x + 7y = 0
(ii) 6x – 3y – 5 = 0
(iii) y=0
Answer:
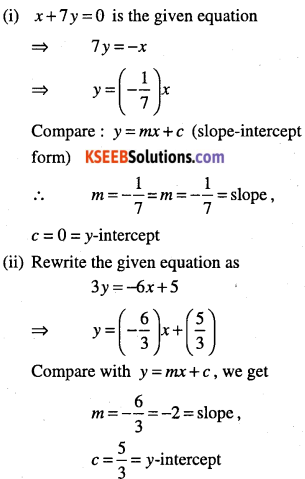
(iii) y = 0
Rewrite as y = (0)x + (0)
Compare with y = mx + c, we get
m = 0 = slope, c = 0 = y – intercept
Question 56.
Reduce the equations into intercept form and find their intercepts on the axes.
(i) 3x + 2y -12 = 0
(ii) 3x – 4y +10 = 0
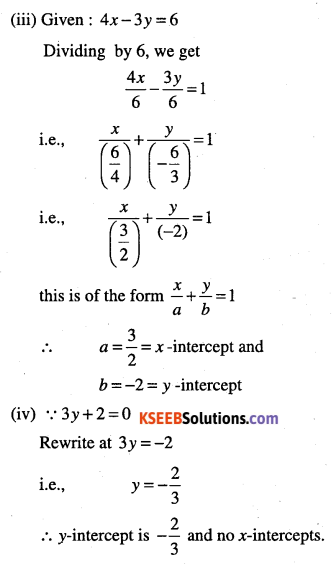
(iii) 4x – 3y = 6
(iv) 3y+ 2 = 0
Answer:
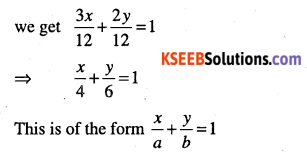
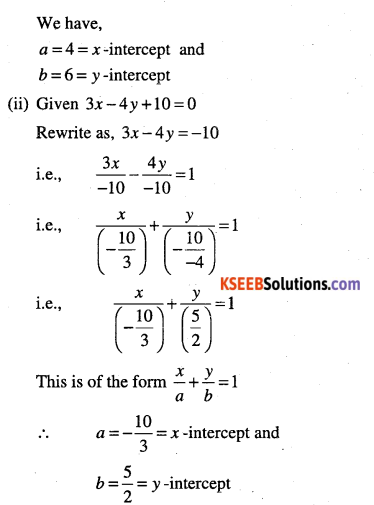
Question 57.
Reduce the equations into normal form, find their perpendicular distances from the origin and angle between perpendicular and the possible x-axis.
(i)\(x-\sqrt{3} y+8=0\)
(ii) y – 2 = 0
(iii) x – y = 4
(iv)\(\sqrt{3} x+y-8=0\)
Answer:

(ii) Given : y – 2 = O
y=2
⇒ o.x +1.y = 2
Compare with x cos(ω) + y sin(ω) = p
we get, cos(ω) = 0, sin(ω) = 1, p = 2
we know that,
cos(90°) = 0 and sin(90°) = 1
ω = 90° and p = 2
∴ Required normal form is,
x cos(90°) + y sin(90°) = 2
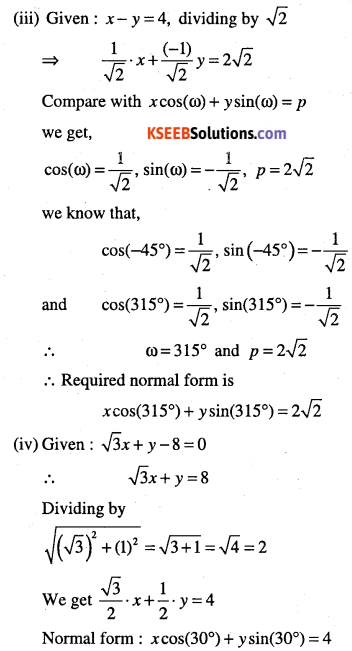
![]()
Question 58.
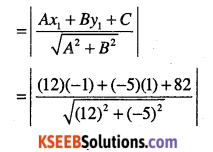
Find the distance of the point (- 1,1) from the line 12(x + 6) = 5 (y – 2).
Answer:
Given equation is,
12(x + 6) = 5(y – 2)
⇒ 12x + 72 = 5m-10
⇒ 12x – 5y + 82 = 0
Compare with Ax + By + C = 0,
we get A = 12, B = -5, C = 82
Given point is (—1,1) = (x1, y1)
∴ Required distance
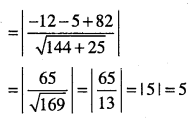
Question 59.
Find the points on the x-axis whose from the line \(\frac{x}{3}+\frac{y}{4}=1\) are 4 units.
Answer:
Rewrite the given equation as 4x ± 3y = 12 or 4x ± 3y – 12 = 0
Any point on the x-axis is of the form (x, 0)
Given that the distance of (x, 0) from the line 4x + 3y – 12 = 0 is 4
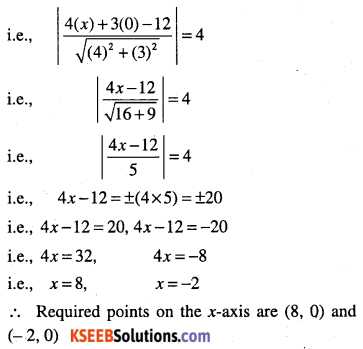
Question 60.
Find the distance between the parallel lines
(i) 15x ± 8y – 34 = 0 and 15x ± 8y ± 31 = 0
(ii) 3x – 4y ± 7 = 0 and 3x – 4y + 5 = 0
(iii) l(x + y) + p = 0 and l(x + y)-r = 0
Answer:
(i) Given equations are
15x ± 8y – 34 = 0 and 15x ± 8y + 31 = 0
Here, A = 15, B = 8, C1 = -34, C2 =31
∴ Required distance is


Question 61.
Find the equation of the line parallel to the line 3x – 4y ± 2 = 0 and passing through the point (- 2,3).
Answer:
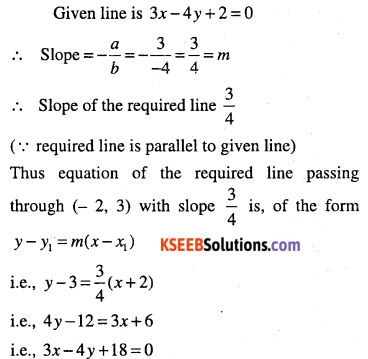
![]()
Question 62.
Find the equation of the line perpendicular to the line x – 7y + 5 = 0 and having x-intercept 3.
Answer:
Given line is x-7y + 5 = 0
\(\text { Slope }=m=-\frac{a}{b}=-\frac{1}{-7}=\frac{1}{7}\)
Slope of the line perpendicular to the given line = – 7 (∵ m1 x m2=-1)
Since x-intercept is 3 then (3, 0) is the point on the required line.
∴ Required equation the line passing through (3, 0) having slope
– 7 is, y – 0 = -7(x-3)
i.e., 7x ± y – 21 = 0
Question 63.
Find angles between the lines \(\sqrt{3} x+y=1\) and \(x+\sqrt{3} y=1\)
Answer:
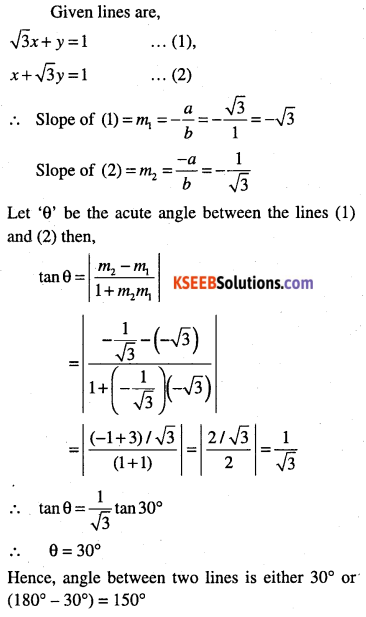
Question 64.
Find the angle between the lines
\(y-\sqrt{3} x-5=0 \text { and } \sqrt{3} y-x+6=0\)
Answer:
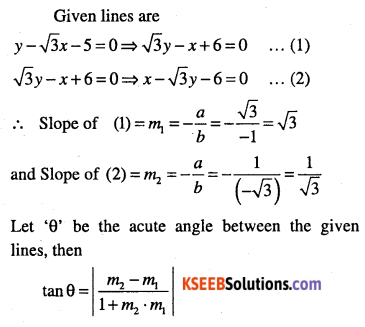
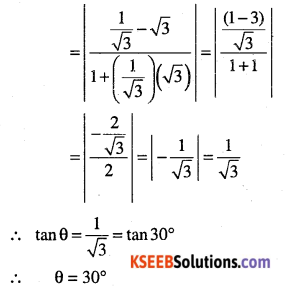
Question 65.
The line through the points (h, 3) and (4,1) intersects the line 7x-9y = 19 at right angle. Find the value of h.
Answer:
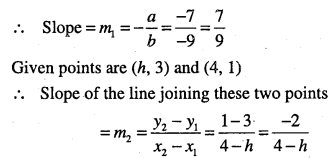
Since the given line and the line passing through the given points are perpendicular to each other, then
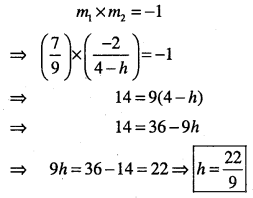
Question 66.
Prove that the line through the point (x1,y1) and parallel to the line Ax + By + C = 0 is
A(x-x1) + B(y-y1) = 0
Answer:
Slope of the given line Ax + By + C = 0 is \(m=-\frac{A}{B}\)
∴ Slope of the line through \(\left(x_{1}, y_{1}\right)=-\frac{A}{B}\)
(Because, the line through (x, y) and given the line are parallel)
Thus, equation of line through (x1,y1) with
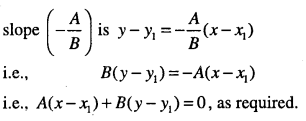
Question 67.
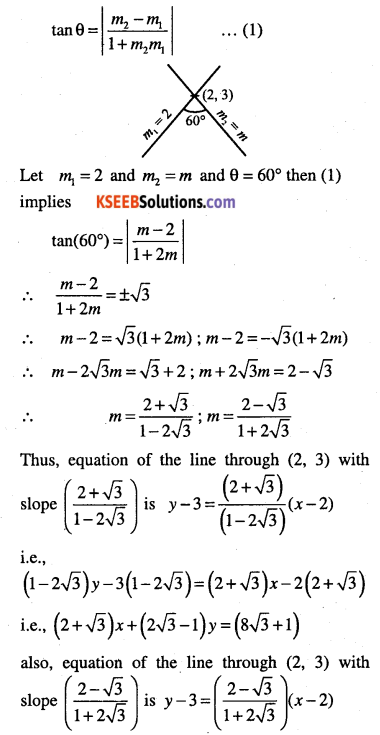
Two lines passing through the point (2, 3) intersects each other at an angle of 60°. If slope of one line is 2, find the equation of the other line.
Answer:
We know that the acute angle ‘0’ between two lines with slopes m1 and m2 is given by

![]()
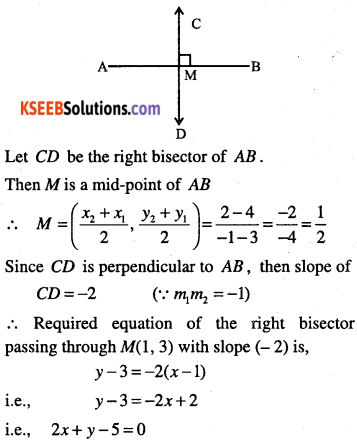
Question 68.
Find the equation of the right bisector of the line segment joining the points (3, 4) and (-1,2).
Answer:
Let A = (3, 4), B = (-1, 2) be the given points.
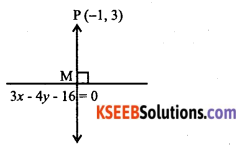
Question 69.
Find the co-ordinates of the foot of perpendicular from the point (- 1, 3) to the line
3x – 4y -16 = 0.
Answer:
Let M be the foot of the perpendicular drawn from p(-1, 3) to the given line P (-1.3)
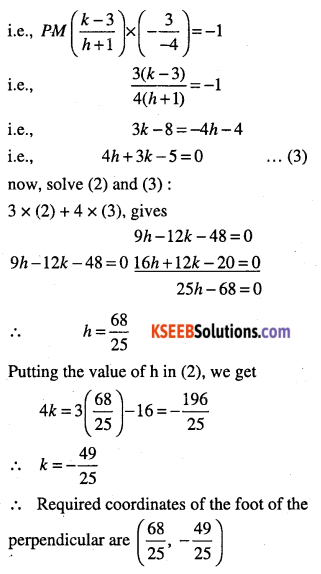
3x – 4y – 16 = 0 ……… (1)
Let the coordinates of M be (h, k)
Since M hes on the line (1), then
3h – 4A – 16 = 0 ………….(2)
Also,
Slope of PM x Slope of (1) = – 1
Question 70.
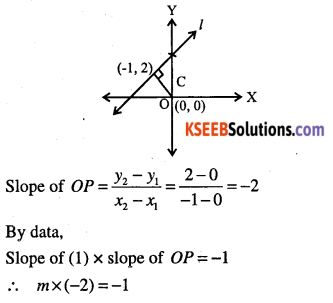
The perpendicular drawn from the origin to the line y = mx + c meets it at the point
(- 1, 2). Find the values of m and c.
Answer:
Given line is y = mx + c ……………. (1)
∵ the point P(-1, 2) lies on (1), then 2 = m(-1) + c
⇒ 2 = – m + c ……………. (2)
Slope of (1) = m and
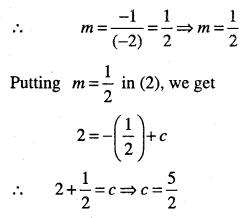
![]()
Question 71.
If p and q are the lengths of perpendiculars from the origin to the lines
x cos θ – y sin θ = k cos 2θ and
x sec θ +y cosec θ = k, respectively, prove that p2 + 4q2 = k2.
Answer:
Given lines are
x cos θ – y sin θ = k cos 2θ …………(1)
x sec θ+y cosec θ = k ……….. (2)
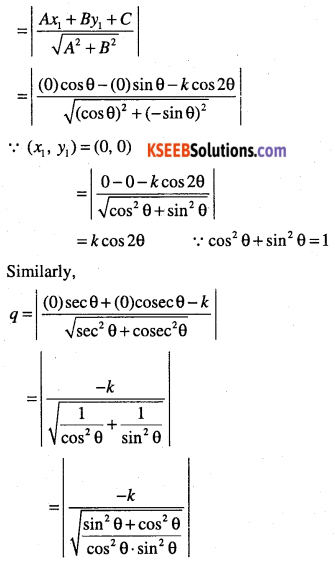
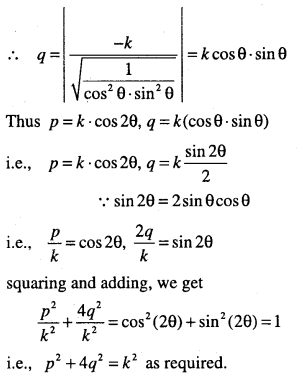
Question 72.
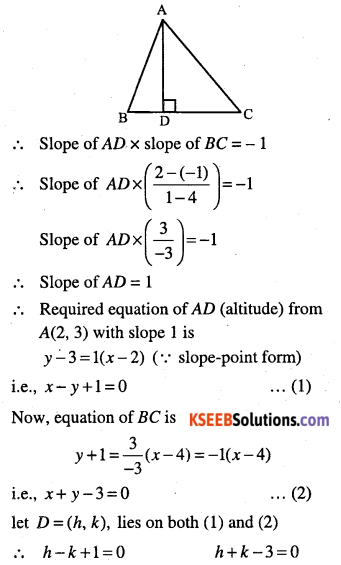
In the triangle ABC with vertices A(2, 3), B(4,-1) and C( 1, 2). Find the equation and length of altitude from the vertex ‘A’
Answer:
A(2,3), B{4,-1) and C(1, 2) be the given points. Let AD be the altitude from A.
∴ AD is perpendicular to BC


Question 73.
If ‘p’ is the length of perpendicular from the origin to the line whose intercepts on the axes area ‘a’ and ‘b’ then show that \(\frac{1}{p^{2}}=\frac{1}{a^{2}}+\frac{1}{b^{2}}\)
Answer:
By intercept form, equation of the line is
\(\frac{x}{a}+\frac{y}{b}=1\)
i.e., bx + ay = ab
i.e., bx + ay-ab- 0
Given :
p = length of perpendicular from (0, 0) to the line
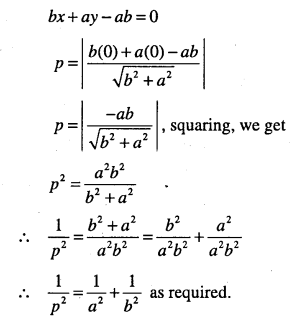
![]()
Miscellaneous examples:
Question 1.
If the lines 2x + y – 3 = 0, 5x + ky – 3 = 0 and 3x – y – 2 = 0 are concurrent, find the value of k.
Answer:
Given lines are

2x + y – 3 = 0 ………….. (1)
5x + ky – 3 = 0 ………….. (2)
3x – y – 2 = 0 …………..(3)
Solve (1) and (3), we get
5x-5 = 0 ⇒ x = 1
and from (1), y = 3-2(1) = 3-2 = 1
∴ x = 1,y = 1
Point of intersection of lines (1) and (3) is (1,1)
Since the given lines are concurrent, the point (1,1) will satisfy (2), so that,
5(1) + k(1)-3 = 0 ⇒ k = -2
Question 2.
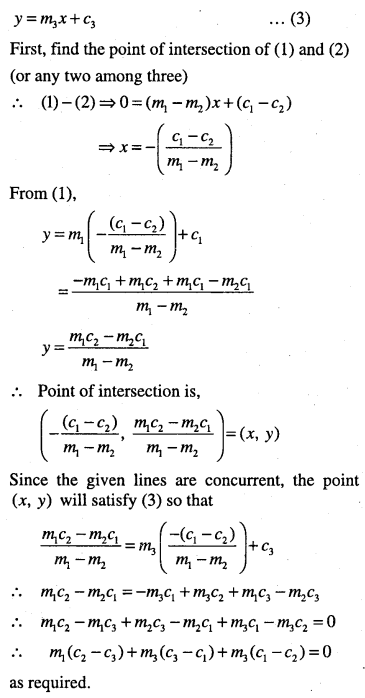
If three lines whose equations are y = m1x + cl, y=m2x + c2 and y=m3 x + c3 are concurrent, then show that m1(c2 – c3)+m2(c3 – c1)+m3(c1 – c2)=O
Answer:

Question 3.
Find the values of k for which the line (k – 3)x – (4-k2)y + k2 -7k+ 6 = 0 is,
(a) parallel to the x-axis
(b) parallel to the y-axis
(c) passing through the origin
Answer:
Give line is,
(k-3)x-(4-k2)y + k2 – 7k+ 6 = 0 …(1)
(a) line (1) is parallel to x-axis if coefficient of x is zero
If coefficient of x is zero
(∵ any line parallel to x-axis is of the form y = b)
k – 3 = 0
⇒ k = 3
(b) Line (1) is parallel to y-axis if coefficient of y is zero
Any line parallel to y-axis is of the form x = a)
∴ 4- k2 = 0
⇒ k = ± 2
(c) Line (1) passes through the origin (0, 0) then
0 – 0 + k2-7k + 6 = 0
i.e., k2 – 7k + 6 = 0
i.e., (k – 6)(k- 1) = 0
⇒ k = 1,6
![]()
Question 4.
Find the value of θ and p, if the equation x cos θ + y sin θ = p is the normal form of the line \( \sqrt{3} x+y+2=0 \)
Answer:
Given Lines

(-cos 30°) + (-sin 30°) y = 1
∴ cos 210° x+sin 210 y=1
Comparing with x cosθ + y sinθ = p
we get
θ = 120°and p = 1
Question 5.
Find the equations of the lines, which cut-off intercepts on the axes whose sum and product are 1 and – 6, respectively.
Answer:
Let a and b be the intercepts of the line on the axes. Then
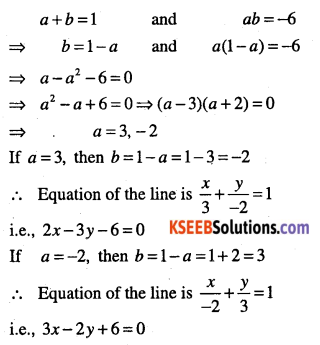
Question 6.
What are the points on the y-axis whose distance from the line \(\frac{x}{3}+\frac{y}{4}=1\)
Answer:

Question 7.
Find the perpendicular distance from the origin of the line joining the points
(cos θ, sin θ) and (cosφ, sinφ).
Answer:
Let A = (cos θ, sin θ) and B = (cosφ, sinφ) be the given points. Then equation of AB is of the form,


Question 8.
Find the equation of the line parallel to y-axis and drawn through the point of intersection of the lines x – 7y + 5 = 0 and 3x + y = 0.
Answer:
Given equations are,
x – 7y + 5 = 0 ……………. (1)
3x + y = 0 ……………. (2)
From (2), y = -3x …………….(3)
Using (3) in (1), we
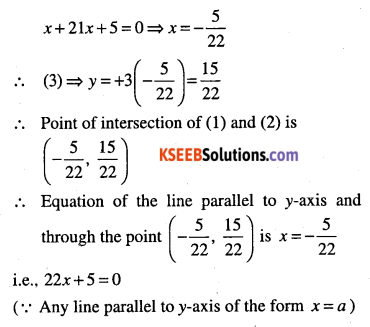
![]()
Question 9.
Find the equation of a line drawn perpendicular to the line \(\frac{x}{4}+\frac{y}{6}=1 \) through the point, where it meets the y-axis.
Answer:
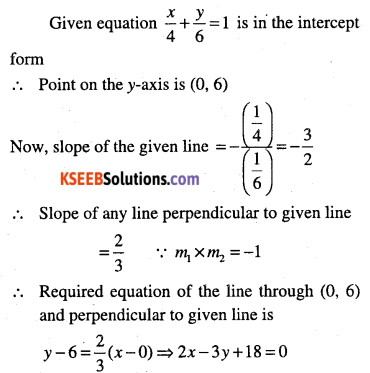
Question 10.
Find the area of the triangle formed by the lines y-x = 0, x + y = 0 and x – k = 0.
Answer: Given lines are
y-x = 0 ………………. (1)
x+ y = 0 …………………….. (2)
First find the points of intersection of the given lines
Solve (1) and (2), we get x = y = 0
Solve (1) and (3), we get x= y =k
Solve (2) and (3), we get x-k, y = -k
∴ Vertices of the triangle are
(0, 0), (k, k) and (k, -k)
∴ Required area

Question 11.
Find the value of p so that the three lines
3x + y – 2 = 0, px + 2y – 3 = 0 and
2x – y – 3 = 0 may intersect at one point.
Answer:
Given lines are,
3x + y – 2 = 0 ………………. (1)
px + 2y – 3 = 0 …………………… (2)
2x – y – 3 = 0 ……………….. (3)
Now, solve (1) and (3): we get
5x – 5 = 0
⇒ x = 1
Using x = 1 on (1), we get y = -1
Point of intersection of (1) and (3) is U,y) = (1,-1)
The three lines are concurrent if the point (1,-1) lies on (2).
i.e., p( 1) + 2(-1)- 3 = 0
⇒ p = 5
Question 12.
Find the equation of the lines through the point (3, 2) which make an angle of 45° with the line x – 2y = 3.
Answer:
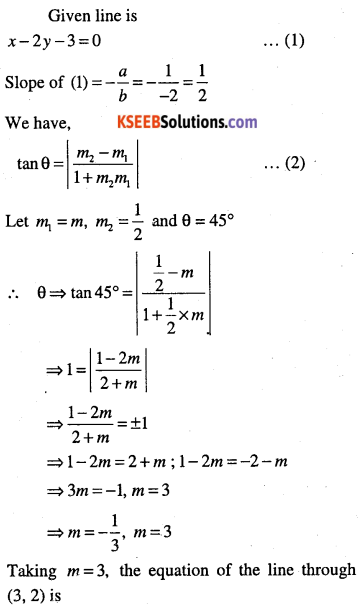

Question 13.
Find the equation of the line passing through the point of intersection of the lines
4 x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes.
Answer:
Given lines are
4x + 1y-3 = 0 …………….(1)
2x – 3y + 1 = 0 ………………. (2)
First, find the point of intersection of (1) and (2),
∴ 3 x (1) + 7 x (2) gives
12 x + 14x – 9 + 7 = 0
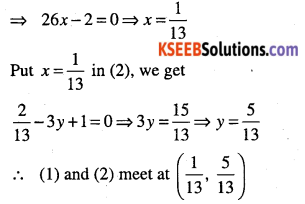
Since the required line has equal intercepts on the axes then its equation is of the form,
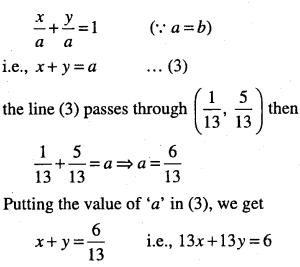
![]()
Question 14.
In what ratio, the line joining (- 1, 1) and (5,7) is divided by the line jc + y = 4?
Answer:
Let A = (-1,1), B(5, 7) be the given points.
Let the given line x+y = 4 divides AB at C in the ratio k : 1
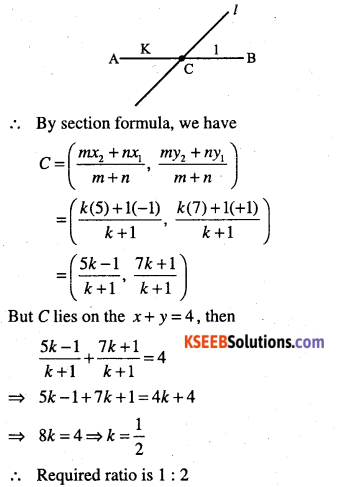
Question 15.
Find the distance of the line 4x + 1y + 5 = 0 from the point (1,2) along the line 2x-y = 0.
Answer:
Given lines are,
4x + 7y +5 = 0 ………..(1)
2x – y = 0 ……………….(2)
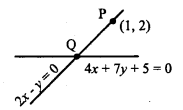
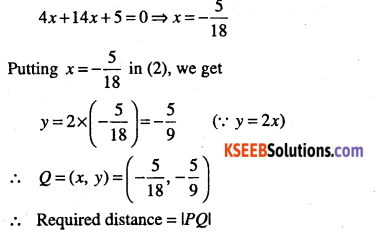

Question 16.
Find the direction in which a straight line must be drawn through the point (- 1, 2) so that is point of intersection with the line x + y = 4 may be at a distance of 3 units from this point.
Answer:
Let the required direction make an angle 0 with the positive direction of x-axis, then the equation of the line through (- 1, 2) and said direction

Required line from (1) is
y – 2 = 0 or + 1 = 0
i.e., Either parallel to x-axis or parallel to y-axis.
Aliter:
Required equation of the line through (x1, y1), making an angle θ with the positive direction x-axis and at a distance r from (x, y) is of the form

Question 17.
The hypotenuse of a right angled triangle has its ends at the points (1, 3) and (- 4, 1). Find the equation of the perpendicular sides (legs) of the triangle.
Answer:
Let P = (1, 3) and Q = (-4,1) be the ends of the hypotenuse.

From the figure, there are two possible right angled triangles PRQ and PSQ.
Clearly R = (1, 1) and 3 = (-4, 3)
Case (i) : In the right angled ΔPQR, required equations are:
Equations to PR and QR
Equation of PR is x = 1 and
Equation of QR is y = 1
Case (ii) : In the right angled ΔPSQ, required equations are:
Equation to PS and QS.
Equation of QS is x = -4 and
Equation of PS is y = 3.
![]()
Question 18.
Find the image of the point (3, 8) with respect to the line x + 3y = 7 assuming that line to be a plane mirror.
Answer:
Let Q = (h, k) be the image of the point P(3, 8) in the line x + 3y-7 = 0 ………… (1)
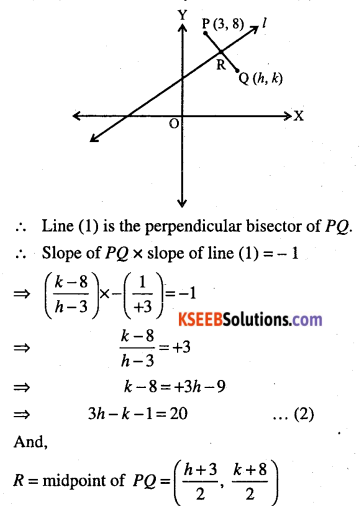
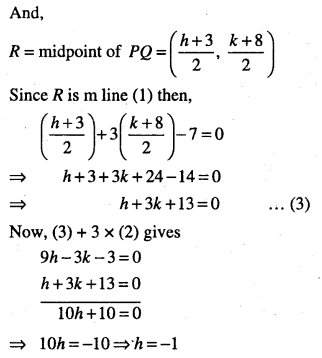
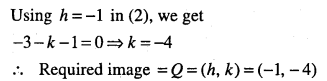
Question 19.
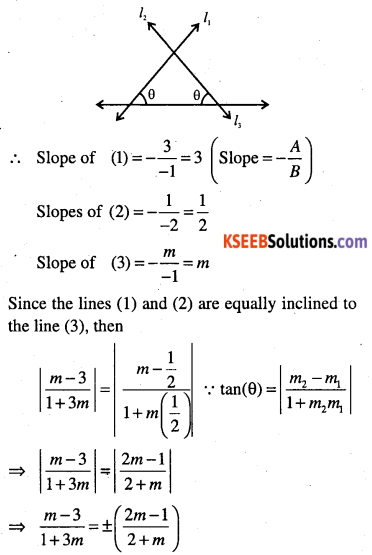
If the lines y = 3x +1 and 2y = x + 3 are equally inclined to the line y = mx + 4, find the value of m.
Answer:
Given lines are
l1 : 3x-y +1 = 0 … (1)
l2: x – 2y + 3 = 0 … (2)
l3: mx – y + u = 0 … (3)
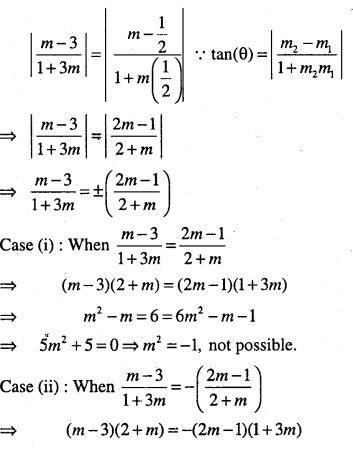
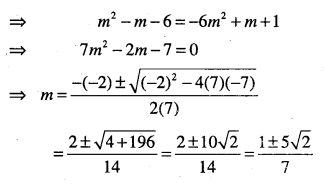
![]()
Question 20.
The sum of the perpendicular distances of a variable point P(x, y) from the lines x + y – 5 = 0 and 3x – 2y + 7 = 0 is always 10. Show that ‘P’ must move on a line.
Answer:
Given lines are
x + y – 5 – 0 ………. (1)
3x – 2y + 7 = 0 …………. (2)
Also,
Distance of (1) from P + distance of (2) from R = 10
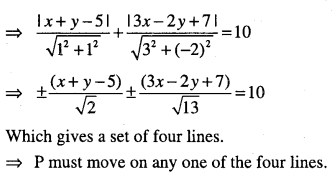
Question 21.
Find equation of the line which is equidistant from parallel lines 9x + 6y – 7 = 0 and
3x + 2y+ 6 = 0.
Answer:
Given lines are
\(3 x+2 y-\frac{7}{3}=0 \quad \ldots(1) \quad(9 x+6 y-7=0)\)
2y + 6 = 0 ……………(2)
Since the required line is equidistant from the parallel lines (1) and (2) then it is of the form,
3x + 2y+ k = 0 …(3)
Given that distance between (1) and (3) equal to distance between (2) and (3).
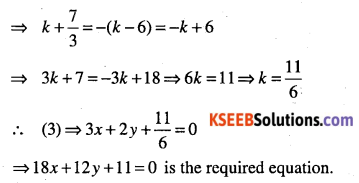
Question 22.
A ray of light passing through the point (1, 2) reflects on the x-axis at point A and the reflected ray passes through the point (5, 3). Find the co-ordinates of A
Answer:
We know that the incident ray and the reflected ray equally inclined to the normal at A. Let A = (h, 0)
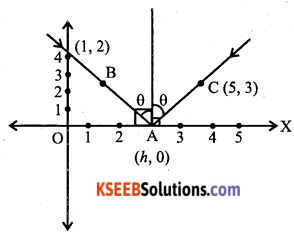
If AC makes an angle (-) with the normal at A, then it makes an angled (90° – θ) with positive x-axis and AB makes an angle (90° + θ) with positive x-axis.
Now, slope of AB = tan (90° + θ) = – cot θ
Slope of AC = tan (90° – θ) = cot θ
∴ Slope of AB + slope of AC = θ
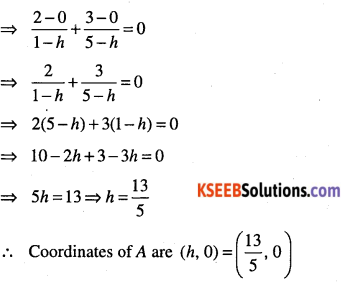
Question 23.
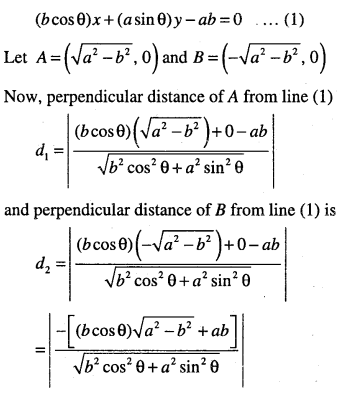
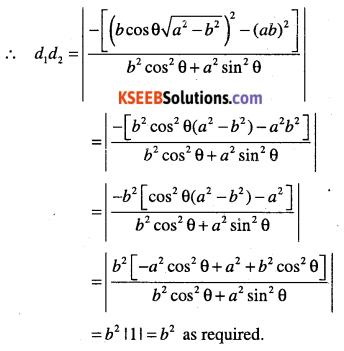
Prove that the product of the lengths of the perpendiculars drawn from the points
\((\sqrt{a^{2}-b^{2}}, 0) \text { and }(-\sqrt{a^{2}-b^{2}}, 0)\) to the line
\(\frac{x}{a} \cos \theta+\frac{y}{b} \sin \theta=1 \text { is } b^{2}\)
Answer:
![]()
Question 24.
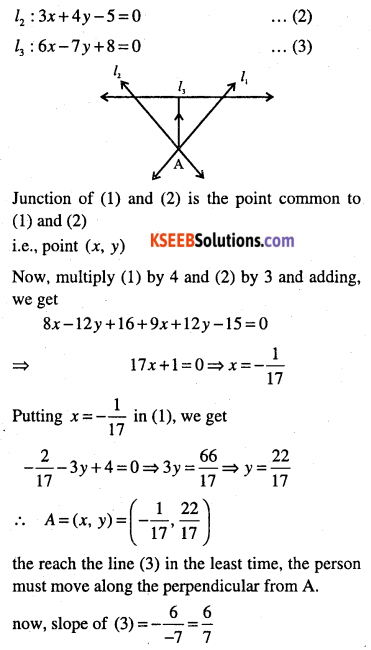
A person standing at the junction (crossing) of the two straight paths represented by the equations 2x – 3y + 4 = 0 and 3x + 4y – 5 = 0 wants to reach the paths whose equation is 6x-7y + 8 = 0 in the least time. Find the equation of the path that he should follow.
Answer:
Given lines are