You can Download Chapter 12 Thermodynamics Questions and Answers, Notes, 1st PUC Physics Question Bank with Answers Karnataka State Board Solutions help you to revise complete Syllabus and score more marks in your examinations.
Karnataka 1st PUC Physics Question Bank Chapter 12 Thermodynamics
1st PUC Physics Thermodynamics Textbook Questions and Answers
Question 1.
A geyser heats water flowing at a rate of 3.0 litres per minute from 27°C to 77°C. If geyser operates on a gas burner, what is the rate consumption of the fuel if the heat of combustion is 4. 0 × 104 J/g
Answer:
Rate of flow of water = 3 litres/minute
∴ Mass of water flowing per minute,
M = 3000g / minute
Initial temperature, T1 = 27°C
Final temperature, T2=77°C
Rise in temperature of the flowing water,
∆ T = T2 – T1 = (77 – 27)° C
= 50° C
specific heat of water, c = 4200 J kg-1 °C-1
= 4.2 Jg-1 °C-1
∴ Total heat supplied by geyser,
∆ Q = mc ∆T
= 3000 × 4.2 × 50
= 630000 J/min
= 6.3 × 105 J/min
Heat of combustion of geyser
= 4 × 104Jg-1
∴ Rate of consumption of fuel,
r = \(\frac{6.3 \times 10^{5}}{4.0 \times 10^{4}}\) = 15. 75 g/min.
Question 2.
What amount of heat must be supplied to 2.0 × 10-2 kg of Nitrogen (at room temperature) to raise its temperature by 45°C at constant pressure? (Molecular mass of N2 is 28; R = 8.3 J mol-1 k-1)
Answer:
Molecular mass of N2 = 28
∴ 1 mole of N2 gas has a mass of 28g.
Given mass of Nitrogen = 2.0 × 10-2 kg
= 20g
∴ Amount of Nitrogen (in moles),
n = \(\frac{20}{28}\) moles = 0. 714.
Molar specific heat capacity at constant
pressure of Nitrogen, Cp = \(\frac{7}{2}\) R
Rise in temperature, ∆ T = 45° C
Total amount of heat to be supplied,
Q = nCp ∆T =0.714 × \(\frac{7}{2}\) × 8.3 × 45
= 933.376 J
Therefore, amount of heat required is 933.376 J.
![]()
Question 3.
Explain why
1. Two bodies at different temperatures T1 and T2 if brought in thermal contact do not necessarily settle to the mean temperature (T1+T2)/2.
2. The coolant in chemical or nuclear plant (i.e, the liquid used to prevent the different parts of a plant from getting too hot) should have high specific heat.
3. Air pressure in car tyre increases during driving.
4. The climate of a harbour town is more temperate than that of a town in a desert at the same latitude.
Answer:
1. When two bodies at different temperatures T1 and T2 are brought in thermal contact, heat energy flows from the body at higher temperature to the body at lower temperature till thermal equilibrium is attained.
Let m be the mass of both bodies and C1 and C2 be the specific heat capacities of bodies at temperatures T1 and T2 respectively. Also, assume that T1> T2.
Since the body at lower temperature absorbs all the energy supplied by a hot body,
∆ Q1 = – ∆ Q2
∴ mc1 ∆T1 = – mc2∆T2 ……..(1)
If ‘T’ is the equilibrium temperature
∴ mc1 (T1 – T) = – mc2(T2 – T) …….(2)
Re-arranging equation (2), we get
T = \(\frac{\mathrm{c}_{1} \mathrm{T}_{1}+\mathrm{C}_{2} \mathrm{T}_{2}}{\mathrm{C}_{1}+\mathrm{C}_{2}}\)
The equilibrium temperature depends on the specific heat capacities of the two bodies and hence cannot be (T1 + T2)/2 in general.
(Note: The common temperature will be (T1 + T2)/2 only if the specific heat capacities are the same, i.e, if C1= C2, then (T1 + T2)/2.
2. The function of a coolant in a chemical or nuclear plant is to absorb as much heat as possible and prevent rise in temperature of the system.
The coolant should have a high specific heat. This is because higher the specific heat of the coolant, higher is its heat-absorbing capacity and vice versa. Hence, a liquid having a high specific heat is the best coolant to be used in a nuclear or chemical plant.
Mathematical analysis:
The equilibrium temperature when two bodies come in thermal contact is given by
T = \(\frac{\mathrm{c}_{1} \mathrm{T}_{1}+\mathrm{c}_{2} \mathrm{T}_{2}}{\mathrm{c}_{1}+\mathrm{C}_{2}}\) ……(1)
where C1, T1, C2, T2 represent specific heat capacity and temperature of first and that of second bodyis respectively.
Let T2<T1, ie the second body is the coolant. Re-writing (1), we have
T = \(\frac{\left(\frac{\mathrm{C}_{1}}{\mathrm{C}_{2}}\right) \mathrm{T}_{1}+\mathrm{T}_{2}}{\left(\frac{\mathrm{C}_{1}}{\mathrm{C}_{2}}\right)+1}\) ……(2)
Since the function of coolant is to keep the temperature of the system constant, the term \(\left(\frac{c_{1}}{c_{2}}\right)\) T1 in equation (2) should be as small as possible. This can be a achieved by having C2>> C1.
Therefore, the specific head capacity of the coolant should be very high.
3. When a car is in motion, the temperature of the tyre rises due to increases in the kinetic energy of the air molecules inside the tyre. Assuming air to be ideal gas, the equation of state is given by
PV = n RT
∴ Change in the state of the system is given by
∆ PV = ∆ n RT ……(1)
Since there is no change in volume or composition, we can rewrite equation (1) as V∆ p = nR ∆ T
Since temperature rises during motion, ∆T is positive. Therefore, ∆P is also positive implying that pressure inside tyre increases when the car is in motion.
4. The humidity in harbour town is generally much greater than humidity in a desert. Since humidity is a measure of water vapor content in the atmosphere and the specific heat of water vapor is very high (≃ 1.86 kJ kg-1 k-1 at 300k) the temperature fluctuations in harbour towns are generally lower than those in desert regions. Hence, the climate in harbour towns is more temperate than that of a town in a desert at the same latitude.
Question 4.
A cylinder with a movable piston contains 3 moles of hydrogen at standard temperature and pressure. The walls of the cylinder are made of a heat insulator, and the piston Is Insulated by having a pile of sand on it. By what factor does the pressure of the gas its original volume?
Answer:
Since the cylinder wall and piston are heat insulators, there is no exchange of heat. Hence, the process is “Adiabatic”.
∴ The equation for the given system is
P1 V1γ = P2 V2γ
where P1 is the Initial pressure of hydrogen in cylinder, V1 isthe initial volume of hydrogen in the cylinder, P2 is the final pressure of hydrogen and V2 is final volume of hydrogen in cylinder, γ is ratio of water specific heat at constant pressure to that at constant volume of hydrogen.
\(\gamma=\left(\frac{\mathrm{C}_{\mathrm{p}}}{\mathrm{C}_{\mathrm{v}}}\right)_{+_{2}} \simeq 1.4\)
∴ \(P_{1} V_{1}^{1.4}=P_{2} V_{2}^{1.4}\)
Since V2 = \(\frac{v_{1}}{2}\), P2=P1 \(\left(\frac{V_{1}}{V_{2}}\right)^{1.4}\)

Thus on decreasing the volume of hydrogen to one half its original value, the pressure increases by a factor of 2.639.
![]()
Question 5.
In changing the state of gas adiabatically from an equilibrium state A to another equilibrium state B, an amount of work equal to 22.3J is done on the system. If the gas is taken from state A to B via a process In which the net heat absorbed by the system is 9.35 cal, how much is the net work done by . the system In the later case ? (Take 1 cal = 4.19 J)
Answer:
Process 1 :
Since the process is adiabatic, net heat supplied to the system is 0. From the first law of thermodynamics, we have
∆ Q = ∆ U + ∆ W ……..(1)
where ∆ Q is the net heat supplied to the system, ∆ U is the change in the internal energy of the gas and ∆ W is the net work done by the gas.
For adiabatic process, ∆ Q = o
∴ From equation (1), ‘
∆ U = – ∆ W
Net work done on the system is 22.3 J
i.e. ∆ w = – 22.3 J
∴ U = – (-22.3) J = 22.3 J.
Process 2 :
Since internal energy, U is a state variable it does not depend on the process but only on the initial and final state of the gas. As the initial and final states for both Process 1 and 2 are A and B respectively, change in internal energy is same as in Process 1.
∴ ∆ U = 22.3 J ………(2)
Net heat absorbed by the system,
∆ U = +9.35 cal =9. 35 × 4.19 J
∴ ∆ Q =39.176 J ……..(3)
From the first law of thermodynamics,
∆ Q = ∆ U + ∆ W
∴ ∆ W = ∆ Q – ∆ U
From equations (2) and (3),
∆ W = 39. 176 – 22. 3 J = 16. 876 J
∴ The network done by the system in the second process is 16. 876 J.
Question 6.
Two cylinders A and B of equal capacity are connected to each other via a stopcock. A contains a gas at standard temperature and pressure. B is completely evacuated. The entire system is thermally Insulated. The stopcock is now opened. Answer the following.
- What is the final pressure of the gas in A and B?
- What is the change in Internal energy of the gas?
- What is the change in temperature of the gas?
- Do the intermediate states of the system (before setting to the final equilibrium state) lie on its P-V-T surface?
Answer:
1. The above process is a case of free expansion of gas. As soon as the stopcock is removed the gas expands to a total volume of twice its original value (as VA = VB).
From Boyle’s law, we have \(P \alpha 1 / v\)
Since V doubles after the stopcock is removed. P reduces to one half the original value.
At STP, pressure of the gas is 100 kPa = 1 Bar
∴ Pressure after expansion = 50 kPa = 0.5 Bar.
2. From the first law of thermodynamics
∆ Q = ∆ U + ∆ W,
Since the process does not involve any work done by the gas such as moving a piston, and no heat is exchanged,
∆ Q = ∆ W = 0
∴ ∆ U = O
∴ There is no change in internal energy of the gas.
3. Since the internal energy of the gas is fixed for the given process, the temperature of the gas also does not change.
ie, ∆ T = ∆ U and if ∆ U = 0, then ∆ T = 0
4. In case of free expansion of gas, the gas does not go through states of thermodynamic equilibrium before reaching the final state. There fore, the thermodynamic parameters such as pressure, volume and temperature are not well defined for these intermediate states and hence, they do not lie on the P-V-T surface for the gas.
Question 7.
A steam engine delivers 5.4 × 108 J of work per minute and services 5.6 × 109 J of heat per minute from its boiler. What Is the efficiency pf the heat engine? How much heat is wasted per minute 7
Answer:
Heat supplied from boiler per minute is 3.6 × 108 J/min
Work done by the steam engine per minute = 5.4 × 108 J/min
Efficiency of steam engine,
\(\eta=\frac{\text { work done }}{\text { heat supplied }}\)
\(\text { i.e. } \eta=\frac{5.4 \times 10^{8}}{3.6 \times 10^{9}}=\frac{5.4}{36}\) = 0.15
∴ Efficiency of the steam engine is 15% Amount of heat wasted per minute
= Heat supplied to steam engine per minute – work done by the engine per minute.
= (3. 6 × 109 – 5.4 × 108) J/ min
= 3. 06 × 109 J/ min
∴ The steam engine wastes 3. 06 × 109 J of energy per minute.
Question 8.
An electric heater supplies heat to a system at a rate of 100 W. If the system performs work at a rate of 75 joules per second, at what rate is the internal energy increasing?
Answer:
Rate of heat supplied to the system is 100 W = 100 Js-1
Rate of work done by the system is 75Js-1.
From the first law of thermodynamics, we have
∆ Q = ∆ U + ∆ W ……..(1)
If the above parameters are observed for a time‘∆ t’,
\(\frac{\Delta \mathrm{Q}}{\Delta \mathrm{t}}=\frac{\Delta \mathrm{U}}{\Delta \mathrm{t}}+\frac{\Delta \mathrm{W}}{\Delta \mathrm{t}}\) …….(2)
where \(\frac{\Delta Q}{\Delta t}\) is the rate of heat supplied to the system for the given time, \(\frac{\Delta U}{\Delta t}\) is the rate of increase in internal energy of the system for the given time and \(\frac{\Delta W}{\Delta t}\) is the
rate of work done by the system in the given time.
∴ From equation (2), we have
100 = \(\frac{\Delta U}{\Delta t}\) + 75
∴ \(\frac{\Delta U}{\Delta t}\) = 25 Js-1
Therefore the internal energy increases at rate of 25 Joules per second.
![]()
Question 9.
A thermodynamic system is taken from an original state D to an Intermediate state E by the linear process shown in Fig. Its volume is then reduced to the original value from E to F by an isobaric process. Calculate the total work done by the gas from D to E to F.
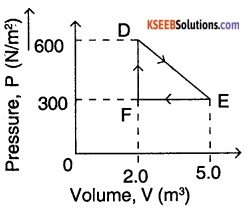
Answer:
In a PV diagram,
1. Work done for a process = Area below the curve for the given process.
∴ For the given PV diagram, work done = Area of ∆ DEF
= \(\frac{1}{2}\) × base × height = \(\frac{1}{2}\) × FE × DF
= \(\frac{1}{2}\) × (5 – 2) × (600 – 300) = \(\frac{1}{2}\) × 3 × 300
= 450J
∴ The total work done by the gas is 450 J.
Question 10.
A refrigerator is to maintain eatables kept Inside at 9°C. If the room temperature is 36°c, calculate the coefficient of performance.
Answer:
Temperature inside the refrigerator,
T1 = 9°C = (9+273) K = 282 K
Room temperature, T2 = 36°C
= (36 + 273) K
= 309 K
The coefficient of performance is,
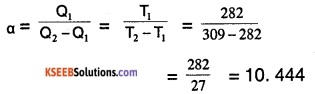
∴ The coefficient of performance of the given refrigerator is 10.444.
1st PUC Physics Thermodynamics One Mark Questions and Answers
Question 1.
What is thermodynamics?
Answer:
Thermodynamics is the branch of physics that deals with interconversion of heat and other forms of energy.
Question 2.
Define thermal equilibrium.
Answer:
A system is said to be in thermal equilibrium if the macroscopic variables that characterise the system to not change whit time.
Question 3.
State zeroth law of thermodynamics.
Answer:
Zeroth law of thermodynamics states that – two systems in thermal equilibrium with a third system separately by are in thermal equilibrium with each other.
![]()
Question 4.
What is the change in internal energy during Isothermal expansion of an ideal gas?
Answer:
Zero, because ∆U α ∆T.
Question 5.
What is an Isotherm?
Answer:
An isotherm is a P – V curve for a fixed temperature.
Question 6.
What is the network done by an ideal gas in an Isochoric process?
Answer:
Zero. Because as the heat absorbed by the gas is entirely used to raise its internal energy.
Question 7.
State the relation between molar specific heat at constant pressure and that at constant volume for an ideal gas.
Answer:
Relation between molar specific heat at constant pressure and that at constant volume for an ideal gas is,
Cp – Cv = R,
where Cp is the molar specific heat at constant pressure, Cv is the molar specific heat at constant volume and R is the Universal gas constant.
Question 8.
What is the network done in a reversible process?
Answer:
Zero. Because, the heat absorbed and change in internal energy are zero.
Question 9.
What is the temperature at which the value in the Celcius scale is equal to that in the Fahrenheit scale?
Answer:
– 40°C
Question 10.
What is refrigeration?
Answer:
The process of transfer of heat from a cold body to a hot body by doing work on the system is called refrigeration.
1st PUC Physics Thermodynamics Two Marks Questions and Answers
Question 1.
State and explain first, law of thermodynamics.
Answer:
The first law of thermodynamics states that the net heat energy supplied to the system is equal to sum of change in internal energy of the system and work done by the system.
Let d Q amount of heat be supplied to the system resulting in a change of internal energy by dU. If the work done by the system is dW then, according to first law of thermodynamics,
dQ = dU + dW
Question 2.
Define molar specific heat at constant pressure, Cp and molar specific heat at constant volume, Cv .
Answer:
Molar specific heat at constant pressure, Cp, is the amount of heat required to raise the temperature of 1 mol of a gas through 1K at constant pressure.
Molar specific heat at constant volume, Cv, is the amount of heat required to raise the temperature of 1 mol of a gas through 1K at constant volume.
Question 3.
A gas is found to obey PV2=Constant. The initial volume and temperature of the gas are Vi and Ti respectively. If the final volume of the gas is Vf, find its final temperature(Tf).
Answer:
We have, P.V2 = constant
i.e. Pi V\(\mathrm{V}_{\mathrm{i}}^{2}\) = Pf V\(\mathrm{V}_{\mathrm{f}}^{2}\) …….(1)
For ideal gas , \(\frac{P V}{T}\) = a constant
\(\therefore \frac{P_{i} V_{i}}{T_{i}}=\frac{P_{f} V_{f}}{T_{f}}\) …….(2)
From equation (2), \(\frac{P_{i}}{f_{i}}=\frac{V_{f}}{T_{f}} \frac{T_{i}}{V_{i}}\) …….(3)
Using equation (3) and (1),
\(\frac{V_{f}}{T_{f}} \cdot \frac{T_{i}}{V_{i}} V_{i}^{2}=V_{f}^{2}\)
⇒ Ti Vi = TfVf or Tf = \(\frac{T_{i} V_{i}}{V_{f}}\)
![]()
Question 4.
Distinguish between isothermal and adiabatic processes.
Answer:
A thermodynamic process in which temperature remains constant is called isothermal process. The change in internal energy for this process is zero. A thermodynamic process in which there is no transfer of heat from system to surroundings is called adiabatic process. The change in internal energy in this process is non-zero.
Question 5.
1 kg of water initially at a temperature 27°C is heated by a heater of power 1KW. If the lid is opened, heat is lost at a constant rate of 200J/S. Find the time required for water to attain a temperature of 80°C with the lid open. (Specific heat of water = 4.2kJ/ kg/k)
Answer:
Amount of water = 1 kg
Net heat supplied = (1000 – 200) = 800 W
Change in temperature = ∆ T
= (353 – 300) = 53K
∴ ∆ T = 53K
Time required, t = \(\frac{\mathrm{H}}{\mathrm{R}}\)
where H is the required energy and R is the rate of heat transfer
H = mC ∆T
= 1 × 4.2 × 103 × 53
= 2.226 × 105 J
R = 8 × 102 J/s
∴ t = \(\frac{2.226 \times 10^{5}}{8 \times 10^{2}}\) = 2.783 × 102
∴ t ≈ 279 = 4 min 39 secs.
Question 6.
Obtain an expression for the coefficient of performance of a refrigerator.
Answer:
A refrigerator transfers heat from a body at lower temperature to a body at higher temperature by doing work on it. If Q2 is the heat absorbed from body at temperature T2 (sink) and Q1 is the heat liberated by the refrigerator to a body at temperature T2 (source) then work done by there refrigerator,
W = Q1 – Q2
∴ The coefficient of performance,
α \(=\frac{Q_{2}}{W}\)
\(=\frac{Q_{2}}{Q_{1}-Q_{2}}\)
\(=\frac{T_{2}}{T_{1}-T_{2}}\)
![]()
Question 7.
The interior of the refrigerator is maintained at 5°C. If the surrounding temperature is 30°C, calculate the coefficient of performance of the refrigerator.
Answer:
The co-efficient of performance of the refrigerator is given by
\(\alpha=\frac{Q_{1}}{w}=\frac{T_{2}}{T_{1}-T_{2}}\)
where T2 is the temperature inside the refrigerator and T1 is the temperature of the surroundings.
∴ \(\alpha=\frac{(30+273)}{\{(30+273)-(5+273)\}}\)
\(=\frac{303}{25}=12.12\)
Question 8.
What is a heat engine? Define efficiency of a heat engine.
Answer:
Heat engine is a device that performs the conversion of heat energy to mechanical work through cyclic process. The efficiency of a heat engine is defined as the ratio of work done by the heat engine to heat absorbed per cycle. If a heat engine absorbs Q1 amount of energy from the source and dissipates Q2 amount of energy to sink, the efficiency η, is given by,
\(\eta=\frac{\mathrm{Q}_{1}-\mathrm{Q}_{2}}{\mathrm{Q}_{1}}=1-\frac{\mathrm{Q}_{2}}{\mathrm{Q}_{1}}\).
Question 9.
The efficiency of a Carnot engine is 50% when the temperature of the sink is 300k, calculate the temperature of the source.
Answer:
For a heat engine, efficiency η, is given by
\(\eta=1-\frac{\mathrm{Q}_{1}}{\mathrm{Q}_{2}}=1-\frac{\mathrm{T}_{2}}{\mathrm{T}_{1}}\)
where, T1 is the temperature of the source and T2 is temperature of the sink ∴
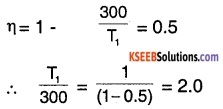
∴ T1 = (300) × (2)
T1 = 600 K
∴ Temperature of the source is 600K.
Question 10.
Prove that for an adiabatic process TVγ-1 constant.
Answer:
We know that for an adiabatic process
PVγ = constant ……(1)
For an ideal gas, \(\frac{P V}{T}\) = constant, C …….(2)
∴ \(P=\frac{C \cdot T}{V}=\) …………(3)
using equation (3) in (1),
\(\left(\frac{C \cdot T}{V}\right) V^{\gamma}\) = constant
i.e. T.Vγ-1 = constant.
1st PUC Physics Thermodynamics Five Marks Questions and Answers
Question 1
Derive the expression for work done by a gas in isothermal process.
Answer:
For an ideal gas,
PV = n RT …….(1)
∴ For isothermal process. T = constant,
work done by an ideal gas,
dW = Pdv ……(2)
where dv is the change in volume of the gas.
using (1) in (2),
\(\mathrm{dW}=\frac{\mathrm{n} \mathrm{RT}}{\mathrm{V}} \mathrm{dv}\)
∴ Total work done,
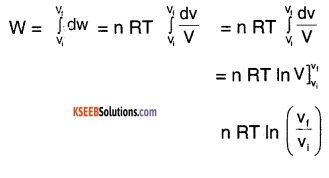
or W = 2.303 n RT log10\(\left(\frac{v_{f}}{v_{i}}\right)\)
Question 2.
Derive an expression for work done by an ideal gas in adiabatic process.
Answer:
Consider n moles of an ideal gas in container such that it is perfectly insulated (thermally) from the surroundings. Let the gas be compressed adiabatically by a small volume, dV.
For adiabatic process of ideal gas,
pvγ = constant ……..(1)
where P is the pressure of the gas, V is the volume of the gas and γ = \(\left(\frac{\mathrm{cp}}{\mathrm{cv}}\right)\) is the ratio of specific heat capacities.
There fore, work done on the gas,
dW = P.dV ……..(2)
From equation (1),
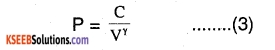
where C is an arbitrary constant.
∴ dW =\(\frac{C}{V^{\gamma}}\).dv
Total work done, W =
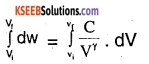
where, Vi is the initial volume of the gas and Vf is the final volume of the gas.

∴ From equation (3),
\(\therefore W=\frac{1}{(1-\gamma)}\left[P_{f} V_{f}-P_{i} V_{i}\right]\) ………..(4)
For an ideal gas,
PV = nRT ….(5)
where n is the no of moles, R is the Universal gas constant and T is the Temperature of the gas.
Using (5) and (4),
\(W=\frac{1}{(1-\gamma)}\left[n R T_{f}-n R T_{i}\right]\)
where Tf is the final temperature and Ti Is the initial temperature
\(\therefore W=\frac{n R}{1-\gamma}\left(T_{t}-T_{1}\right)\)
![]()
Question 3.
What is Carnot engine? Describe the different parts of the Carnot heat engine.
Answer:
Carnot engine is an ideal heat engine which has ideal gas as working substance.
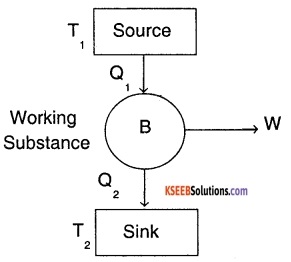
The above diagram shows the working of a carnot heat engine. It consists of the following parts:
1. Source :
It is a source of heat maintained at constant temperature T1
2. Sink:
It is a sink for heat maintained at constant temperature T2.
3. Working substance:
Ideal gas contained in a cylinder with perfectly non-conducting walls and perfectly conducting bases.
Question 4.
Describe the working of Carnot engine and mention the expression for its efficiency.
Answer:
A carnot engine is a reversible heat engine operating between two temperatures,
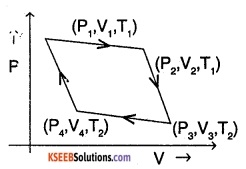
The steps involved in a carnot cycle are explained as follows.
Step 1:
Isothermal expansion of gas from state (P1, V1, T1) to state (P2, V2, T1) as shown in the figure.
Step 2:
Adiabatic expansion of gas from (P2, V2, T1) to (P3, V3, T2)
step 3:
Isothermal compression of gas from (P3, V3, T2) to (P4, V4, T2)
step 4:
Adiabatic compression of gas from (P4, V4, T2) to (P1, V1, T1)
These steps constitute one carnot cycle. The process is repeated thereafter.
The efficiency of carnot engine, η is given by
\(\eta=\frac{\mathrm{Q}_{1}-\mathrm{Q}_{2}}{\mathrm{Q}_{1}}=\frac{\mathrm{T}_{1}-\mathrm{T}_{2}}{\mathrm{T}_{1}}\)
\(\therefore \eta=1-\frac{T_{2}}{T_{1}}\)
1st PUC Physics Thermodynamics Numerical Problems Questions and Answers
Question 1.
Two moles of an ideal gas undergoes Isothermal expansion when heat is supplied to It. If the gas is at room temperature (298K) and the final volume is thrice the Initial volume, find the total heat supplied.
Answer:
No of moles, n = 2
temperature, T = 298K
let initial volume = Vi
∴ final volume, Vf = 3Vi
The work done for isothermal process is given by,
W = 2.303 nRT log10 \(\left(\frac{v_{2}}{v_{1}}\right)\)
where V2 is the final volume and V1 is the initial volume.
Here \(\frac{v_{2}}{v_{1}}\) = \(\frac{v_{f}}{v_{i}}=3\)
∴ W = 2.303 × 2 × 8.314 × 298 × \(\log _{10}^{3}\)
W = 5.44kJ
Since, for isothermal process, Heat supplied is equal to work done by the gas, the total heat supplied is 5.44 kJ.
Question 2.
An Ideal gas under goes adiabatic expansion to twice original volume. If the gas was initially at 40°C, calculate its temperature after the process.
[Take 1 = \(\frac{5}{3}\)]
Answer:
Let the initial volume, V = V0
Final volume, V = 2.V0
Initial temperature, Ti = 40°C
= 40 + 273
= 313K
Let final temperature = Tf
For an adiabatic process,
TVγ-1 = constant
∴ Ti \(V_{i}^{\gamma-1}\) = Tf \(V_{f}^{\gamma-1}\)
∴(3/3).\(\left(V_{0}\right)^{\frac{5}{3}-1}\) =Tf \(\left(2 V_{0}\right)^{\frac{5}{3}-1}\)
∴ \(\mathrm{T}_{\mathrm{f}}=\frac{3 / 3}{2^{\frac{2}{3}}}\)
∴ Tf = 197.18 K
Therefore, on adiabatic expansion, the temperature of the gas drops to 197.18 K.
Question 3.
One mole of an ideal gas is supplied 2KJ of heat. If the temperature of the gas rises from 0°C to 200°C, calculate work done by the gas and change in its internal energy if
- The process Is isobaric
- The process is isochoric.
Answer:
number of moles, n = 1
Initial temperature, Ti = (0 + 273)
= 273 K
Final temperature, Tf = (200 + 273)
= 473 K
Net heat supplied, H = 2000 J
1. Isobaric process :
work done, W = P – ∆ V
or W = n R∆T
∴ W= 1 × 8.314 × (473-273)
∴ W= 1662.8J = 1.66KJ
∴ Change in internal energy,
∆U = H – W= 2000 – 1662.8
= 337.2J
2. isochoric Process:
Work done in an isochoric process , W =0. Therefore change in internal energy
∆U = H = 2 kJ
Question 4.
One mole of an ideal gas undergoes a cyclic process as shown in the figure.
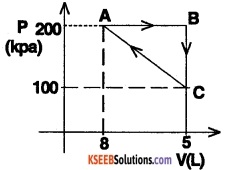
Calculate the work done by the gas in each of the process. A-B, B-C, and C-A. What is the network done by the gas at the end of one cycle?
Answer:
1. The process A – B is isobaric. Therefore work done is given by,
WAB = P. ∆V
= 200 × 103 × (5-3) × 10-3
= 400J
∴ Also, work done is given by area under the curve, AB.
Area = (AB) × (200) × 103
= (2 × 10-3) × 200 × 103 =400
∴ Area = WAB.
2. Work done in the process BC,
WBC = Area under the curve BC = 0
3. Work done in process CA,
WCA= – (Area under the curve CA)
∴ WCA = – [ \(\frac{1}{2}\) × (100 + 200) × 103 × (2 × 10-3)]
∴ WCA = – 300 J
∴ Total work done by the gas at the end of cycle,
Wtotal = (400 + 0 – 300) J = 100 J
Question 5.
Two samples A and B of same gas have same Initial pressure and volume. A undergoes an Isothermal expansion and B is subjected to adiabatic expansion. If the final volume in both cases is double the initial volume and work done In both the cases are same, then prove that
![]()
Answer:
Let the initial pressure, pi = p1
initial volume, Vi = V1
final volume, Vf = V2
= 2V1
Work done in isothermal process,
Wiso = 2.303 RT log \(\left(\frac{V_{2}}{V_{1}}\right)\)
Since PV = RT (1 mol of gas),
Wiso = P1 V1 ln2 ……(1)
work done in adiabatic process,
wad = \(\frac{1}{\gamma-1}\) [P1 V1 – P2 V2]
\(=\frac{P_{1} V_{1}}{\gamma-1}\left[1-\frac{P_{2} V_{2}}{P_{1} V_{1}}\right]\)
For adiabatic process,
P1 \(V_{1}^{\gamma}\) = P1 \(V_{2}^{\gamma}\) = K (constant)

Since work done is same for both processes,
Wiso = Wad
∴ \(P_{1} V_{1} \ln 2=\frac{P_{1} V_{1}}{\gamma-1}\left(1-2^{1-\gamma}\right)\) ……….(3)
Re-arranging and simplifying (3),
21-γ – 1 = (1 – γ) ln2.
![]()
Question 6.
Two moles of a monoatomic gas is taken through a cyclic process starting from A as shown in the figure. The
volume ratios are \(\frac{v_{B}}{V_{A}}\) =2 and \(\frac{v_{D}}{V_{A}}\)= 4. If the temperature TA at A is 27°C, calculate,
- Temperature of the gas at point B
- heat absorbed or released by the gas in each process
- the total work done by the gas during the complete cycle.
Express your answer In terms of gas constant R.
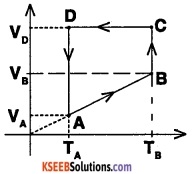
Answer:
1. From the figure, for the process A – B, we have
V = k. T
where V = Volume of the gas
T = Temperature of the gas
k = proportionality constant
∴ \(\frac{\mathrm{v}}{\mathrm{T}}\) = k
∴ \(\frac{V_{A}}{T_{A}}=\frac{V_{B}}{T_{B}}\) and \(\frac{V_{B}}{V_{A}}=\frac{T_{B}}{T_{A}}\)
∴ TB = TA × 2
TB = (27 + 273) × 2 = 600K
2. Process A – B :
HAB = nCp∆T
HAB = 2 × [\(\frac{5}{2}\) R] × (600 – 300)
HAB = 1500 R (Heat absorbed)
3. Process B – C:
HBC = 2.303nRTB log \(\frac{V_{D}}{V_{B}}\)
VD = 4VA
VB = 2VA
∴ \(\frac{V_{D}}{V_{B}}\) = 2
∴ HBC = 2.303 × R × 600 × log 2
∴ HBC ≃ 831.6 R
4. Process C – P :
HCD = nCv ∆T
HCD = 2 × [\(\frac{3}{2}\) R] × (-300)
∴ HCD = – 900 R
5. Process D – A :
HDA = 2.303 × nRTA × log \(\frac{V_{A}}{V_{D}}\)
∴ HDA = 2.303 × 2 × R × 300 × log 4
∴ HDA ≃ – 831.6 R
(3) From first law of thermodynamics,
∆H = ∆U + ∆W
where,
∆ H = net heat supplied to the system
∆ U = Net change in internal energy and
∆W = total work done by the system.
For a cyclic process, ∆U = 0
∴ ∆H = ∆W
∴ Total work done by the system,
∆W = HAB + HBC + HCD + HCA
= 1500 R + 831.6 R – 900 R – 831.6R
∴ ∆W = 600 R
Therefore, total work done by 2 mols of the given monoatomic gas is 600 R.
Question 7.
One mole of an Ideal gas Initially at 300K Js expanded Isothermally so that Its volume increases 5 times. It is then heated at constant volume to restore initial pressure. If the net heat supplied Is 29KJ find γ for the given gas.
Answer:
Total heat supplied,
Htot = 2.303RT1log\(\frac{V_{2}}{V_{1}}\) + Cv ∆T
= 2.303 × 8.314 × 300 × log5 + \(\frac{C_{v}}{R}\) (R∆T)
Here, Htot = 29000 J
R∆T = ∆PV = P3 V3 – P2 V2
P3 = P1
V3 = 5.V1 = V2
P2 = \(\frac{P_{1}}{5}\)
∴ ∆PV = P1.5V1 – \(\frac{P_{1}}{5}\) . 5V1
=4 P1 V1 = 4. R. T1
\(\therefore \frac{C_{v}}{R}=\left(\frac{29000-2.303 \times 8.314 \times 300 \times \log 5}{4 \times 8.314 \times 300}\right)\)
∴ \(\frac{C_{v}}{R}\) = 2.505
∴ Cv ≃ 2.5R
∴ Cp ≃ 3.5R (Cp = Cv + R)
∴ γ = \(\frac{C_{p}}{C_{v}}=\frac{3.5}{2.5}=1.4\)
Question 8.
A Carnot engine operates between 900K and 300K.The efficiency of the engine has to be increased to 80%
- By how much should the temperature of the source alone be increased?
- By how much should the temperature of the sink alone be lowered?
Answer:
Efficiency of a carnot engine is given by,
\(\eta=1-\frac{T_{2}}{T_{1}}\)
where T2 = Temperature of the sink
T1 = Temperature of the source
1. 0.8 = 1 – \(\frac{300}{T_{1}}\)
∴ \(T_{1}=\frac{300}{1-0.8}=\frac{300}{0.2}=1500 \mathrm{K}\)
∴ Temperature of the source alone has to be increased by 600K.
2. 0.8 = 1 – \(\frac{T_{2}}{900}\)
∴ T1 = 900 (1-0.8) = 180 K
∆T = 300-180 = 120K
Therfore temperature of the sink alone has to be lowered by 120K.
Question 9.
The efficiency of a Carnot’s engine is 20%. When the temperature of the sink Is reduced by 100°C, efficiency increases to 40%. Find the initial temperature of the source and sink.
Answer:
Let the initial temperature of source, T1i = T1 and that of the sink be T2i = T2
∴Final temperature of sink, T2f = T2 – 100
Efficiency of the Carnots engine is given T2
by, \(\eta=1-\frac{T_{2}}{T_{1}}\)
where, T2 = Temperature of sink
T1 = Temperature of source
∴ 0.2 = 1- \(\frac{T_{2}}{T_{1}}\) …….(1)
\(0.4=1-\frac{\left(T_{2}-100\right)}{T_{1}}\) ………..(2)
Re – arranging equation (2),
\(0.4=1-\frac{T_{2}}{T_{1}}+\frac{100}{T_{1}}\) …….(3)
Using equation (1) in equation (3),
\(0.4=0.2+\frac{100}{T_{1}}\)
∴ \(\mathrm{T}_{1}=\frac{100}{0.4-0.2}=500 \mathrm{K}\)
On substituting in (1) we have,
\(0.2=1-\frac{100}{T_{1}}\)
∴ T2 = 500 (1 – 0.2)
= 400 K
∴ Initial temperatures of source and sink are respectively 500K and 400 K.
![]()
Question 10.
- Two Carnot engines are working between 400K and 300K, 300K and 200K respectively. Find the ratio of the efficiency of first system to the second system.
- If 200 J of heat is supplied to a carnot engine at 400K and 50J of work Is done by the system, find the temperature of the sink and efficiency of the engine.
Answer:
1. System 1:
Temperature of source, T1 = 400K
Temperature of sink, T2 = 300K
∴ efficiency of the engine is,
\(\eta_{1}=1-\frac{T_{2}}{T_{1}}=1-\frac{300}{400}=0.25\)
System 2:
Temperature of source, T1 = 300K
Temperature of sink, T2 = 200K
∴ efficiency of this engine is,
\(\eta_{2}=1-\frac{T_{2}}{T_{1}}=1-\frac{200}{300}=0.33\)
∴ Ratio of efficiencies is,
\(\frac{\eta_{1}}{\eta_{2}}=\frac{0.25}{0.33}=\frac{1 / 4}{1 / 3}=\frac{3}{4}=0.75\)
2. Temperature of source, T1 = 400 K
Hear supplied to engine, Q1 = 200 J
Work done by the engine, W = 50 J
∴ Heat rejected of the engine,
Q2 = Q1 – W = 150 J
∴ efficiency of the engine is,
\(\eta=1-\frac{Q_{2}}{Q_{1}}=1-\frac{150}{200}=1-\frac{3}{4}=0.25\)
Let temperature of sink, T2 = T
∴ \(\eta=1-\frac{T_{2}}{T_{1}}=1-\frac{T}{T_{1}}\)
∴ T = T1 (1 – η) = 400 (1 – 0.25)
= 300 K
Question 11.
A carnot engine has an efficiency of \(\frac{1}{2}\). On increasing the temperature of the sink by 100°C, the efficiency drops to \(\frac{1}{3}\). Find the temperature of the source and sink in the original state. By what amount should the source temperature be increased to restore the original efficiency?
Answer:
Let temperature of source = T1
let temperature of sink = T2
∴ efficiency of the engine, \(\eta=1-\frac{T_{2}}{T_{1}}\)
\(\therefore 1-\frac{T_{2}}{T_{1}}=\frac{1}{2}\) ……(1)
on increasing T2 by 100°C, efficiency,
\(\eta=1-\frac{\left(T_{2}+100\right)}{T_{1}}=\frac{1}{3}\)
\(\therefore\left(1-\frac{T_{2}}{T_{1}}\right)-\frac{100}{T_{1}}=\frac{1}{3}\) …..(2)
substituting (1) in (2),

Thus initial temperature of source and sink are 600K and 300 K respectively After raising temperature of sink,
T2f = 300 + 100 =400 K
To restore original efficiency \(\left(\eta=\frac{1}{2}\right)\) let the source temperature be raised by ‘T’ K.

Therefore, the temperature of the source should be raised by 200 K to restore original efficiency.