2nd PUC Maths Question Bank Chapter 9 Differential Equations Ex 9.3

Students can Download Maths Chapter 9 Differential Equations Ex 9.3 Questions and Answers, Notes Pdf, 2nd PUC Maths Question Bank with Answers helps you to revise the complete Karnataka State Board Syllabus and score more marks in your examinations.

Karnataka 2nd PUC Maths Question Bank Chapter 9 Differential Equations Ex 9.3

2nd PUC Maths Differential Equations NCERT Text Book Questions and Answers Ex 9.3

In each of the Exercises 1 to 5, form a differential equation representing the given family of curves by eliminating arbitrary constant a and b.

Question 1.
\(\frac{x}{a}+\frac{y}{b}=1\)
Answer:

Question 2.
y² = a (b² – x²)
Answer:
y² = a (b² – x²)
Differentiating w.r.t x, we get
\(2 y \frac{d y}{d x}=a(0-2 x) \Rightarrow 2 y y_{1}=-2 a x\)
=y y, = -ax ………(1)
differentiating again
yy₂ + yy₁ = -a …(2)
putting value of (-a) from (2) in (1)
yy₁= (yy₂ + y₁y₁) x
⇒ y y, = x (y₁² + yy₂) which is required differential equation.
Note:-
Try to form differential equation with lowest order by removing all arbitrary constants

Question 3.
y = a e^3x + b e^-2x
Answer:
y = a e^3x + b e^_2x ….(1)
Differentiating w.r.t x , y, = ae^3x.3 + be ^2x (-2)     ….(2)
multiply (1) by 3
⇒ 3y = 3ae^3x + 3be^_2x
⇒ 3ae^3x = 3y -3be^-2x …(3)
put (3) in (2), we get y, = 3y – 3be^-2x – 2 be^-2x
⇒ y – 3y = -5 be^-2x …….. (4)
differentiating again
⇒ y₂ – 3y₁ = -5 be^-2x (-2) ….(5)
multiply (4) by 2, we get
⇒ 2y,-6y = -10be ^2x ….(6)
putting (6) in (5), we get
⇒ -(2y₁ – 6y) = y₂ -3y₁
⇒ y₂ – 3y₁ + 2 (y₁ 3y) = 0
⇒ y₂ -y_t – 6y = 0 is the required differential equation.

Question 4.
y = e^2x (a + bx)
Answer:
y = e^2x (a + bx) …(1)
differentiating w.r.t x, we get
⇒ y₁= e^2x (0 + b) + (a + bx) e^2x 2
⇒ y₁ = e^2xxb + (a + bx) e^2x 2      ….(2)
putting (1) in (2),
y₁ = be^2x + 2y ⇒ y₁ – 2y = b e^2x ….(3)
again differentiating w.r.t x, we get
y₂ – 2y₁ = b.e^2x.2 ….(4)
putting (3) in (4) we get
y₂ – 2y₁ = ²y₁ – 4y
⇒ y₂ – 4y₁ + 4y = 0 is
the required differential equation.

Question 5.
y = e^x (a cos x + b sin x)
Answer:
y₁ = e^x (a cos x + b sin x) ….(1)
differentiate w.r.t x
y₁ = e^x (a cos x + b sin x) + e^x (-a sin x + b cos x) ….(2)
putting (1) in (2); we get
⇒ y₁ = y + e^x (b cos x – a sin x)
⇒ y₁ – y = e^x (b cos x – a sin x) ….(3)
again differentiating w.r.t x, we get
⇒ y₂ – y₁ = e^x (b cos x – a sin x) +e^x (-b sin x – a cos x) ….(5)
putting (3) and (1) in (5), we get
⇒ y₂ – y₁ = (y₁ – y) +(-y)
⇒ y₂ – y₁ + 2y = 0 is the required differential equation.

Question 6.
Form the differential equation of the family of circles touching the y-axis at origin.
Answer:
Any circle that touches ‘y’ axis at origin should have centre on ‘x’ axis and pass through origin. If centre is (h, 0) then radius is distance between (h, 0) and (0,0), so radius
\(=\sqrt{(h-0)^{2}+(0-0)^{2}}=h\)
Equation of circle
= (x – h)² + (y – 0)² = radius² = h²
⇒ x² + h² – 2hx + y² = h²
⇒ x²-2hx + y² = 0         …(1)
differentiating w.r.t x, we get
⇒ 2x + 2y y, – 2h = 0  …(2)
multiply (2) with x and subtracting from (1), we get
⇒2x² + 2y y₁ x – 2hx – x² + 2hx – y² = 0
⇒ x² – y² + 2y y₁ x = 0 is the required differential equation.

Question 7.
Form the differential equation of the family of parabolas having vertex at origin and axis along positive y-axis.
Answer:
Equation of parabola required in question is:-
x² = 4 ay ………… (1)
a is parameter differentiating w.r.t x, we get
2x = 4ay ……….. (2)
putting (1) in (2), we get x²
\(2x=\frac{x^{2}}{y} y_{1} \Rightarrow 2y=x y_{1} \Rightarrow x y_{1}-2 y=0\)
required differential equation.

Question 8.
Form the differential equation of the family of ellipses having foci on y-axis and centre at origin.
Answer:
Equation of ellipse required in question is
\(\frac{x^{2}}{b^{2}}+\frac{y^{2}}{a^{2}}=1\)
a=semi major axes (along ‘y’axis)
b = semi minor axes differentiating w.r.t x, we get

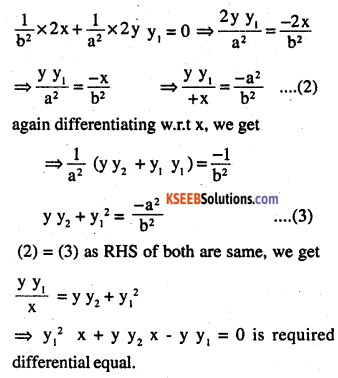
Question 9.
Form the differential equation of the family of hyperbolas having foci on x-axis and centre at origin.
Answer:
Equation of hyperbola required in question is:-

Question 10.
Form the differential equation of the family of circles having centre on y-axis and radius 3 units.
Answer:
Required equation of circle is :-
(x – 0)² + (y – k)² = 3² ……….(1)
(k is any value) – as along ‘y’ axis, centre will be (0, k)

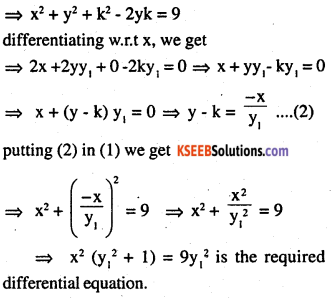
Question 11.
Which of the following differential equations has y = c₁ e^x + c₂ e^-x as the general solution?

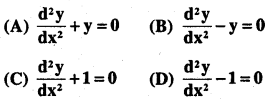
Answer:

but (1) = (3)
⇒ y = y₂
so y₂ – y = 0
so correct answer is (B).

Question 12.
Which of the following differential equations has y = x as one of its particular solution ?

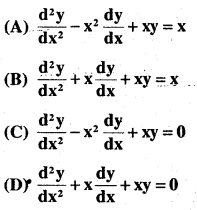
Answer:
y = x
y₁ = 1 y₂ = o
this is satisfied by
y₂ – x² y, + xy = 0
0 – x² x 1 + x x x = 0
so answer is (C).