Chapterwise 1st PUC Statistics textbook solutions karnataka Board are given here in PDF format for understanding the concepts involved in the syllabus. If you are looking for Chapter-wise KSEEB Solutions for 1st PUC Statistics Question Bank with Answers Solutions, Notes, Guide Pdf are given in Chapter wise format then this is the right page for you. Students are advised to download chapter wise 1st PUC Question Bank with Answers Karnataka solutions pdf to take their preparation to the next level. Go through this page and get all chapters pdf links of KSEEB solutions for 1st PUC Statistics textbook solutions pdf & learn efficiently to score high marks.
Students can also read 1st PUC Statistics Model Question Papers with Answers hope will definitely help for your board exams.

Karnataka 1st PUC Statistics Question Bank with Answers
By taking the help of the best resources like Karnataka State Board Solutions for 1st PUC Statistics Textbook Questions and Answers you all can easily understand the concepts involved in the latest KTBS Solutions are given in syllabus and gain more subject knowledge. Study 1st PUC Statistics Question Bank with Answers Karnataka solutions on a daily basis and be confident to answer all the questions asked in the final exam. Chapter-wise KSEEB solutions for 1st PUC Statistics PDF links are available below to download.
- Chapter 1 Introduction – Meaning and Scope
- Chapter 2 Organization of Data
- Chapter 3 Classification and Tabulation
- Chapter 4 Diagrammatic and Graphical Presentation of Data
- Chapter 5 Analysis of Univariate Data
- Chapter 6 Analysis of Bivariate Correlation and Regression
- Chapter 7 Associates of Attributes
- Chapter 8 Interpolation and Extrapolation
- Chapter 9 Elements of Probability Theory
- Chapter 10 Random Variables & Mathematical Expectation
1st PUC Statistics Features of the Question Bank
- For the first time Pre-University Department has been released the Question Bank for the First Year PUC Statistics.
- First Year PUC Statistics Text Book contains 10 units
- The questions in the Question Bank are framed for all the units on the basis of the text book.
- Following is the pattern of the Question Bank.
Section A-each question carries one mark.
Section B – each question carries two marks.
Section C – each question carries five marks.
Section D- each question carries ten marks.
Section E- each question carries five marks (Practical – oriented questions). - Tests, Mid-term and Annual Examination Question Papers should be based on this Question Bank.
- Model Question Papers are given at the end of the question bank.
Karnataka 1st PUC Statistics Question Paper Pattern
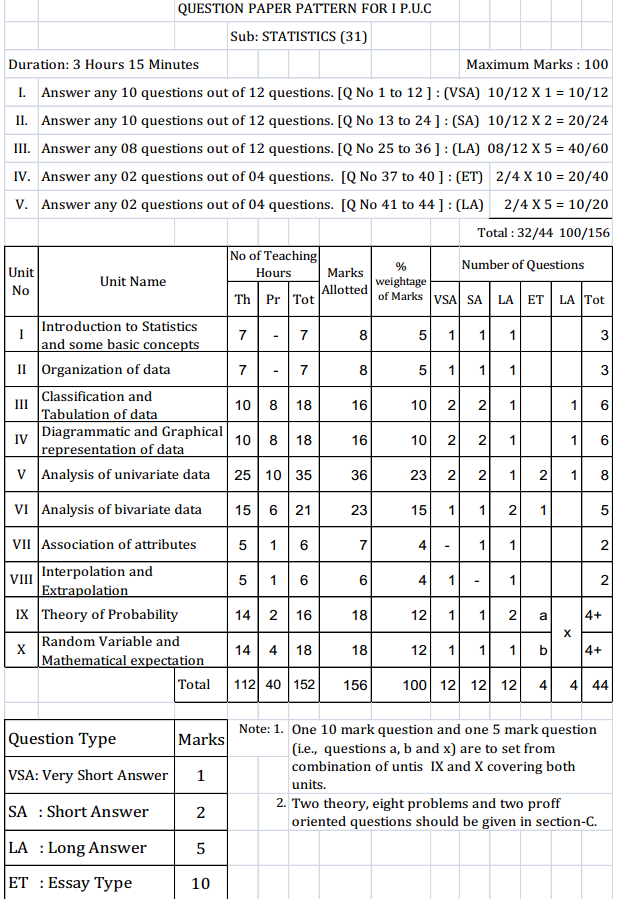
Karnataka 1st PUC Statistics Blue Print of Model Question Paper
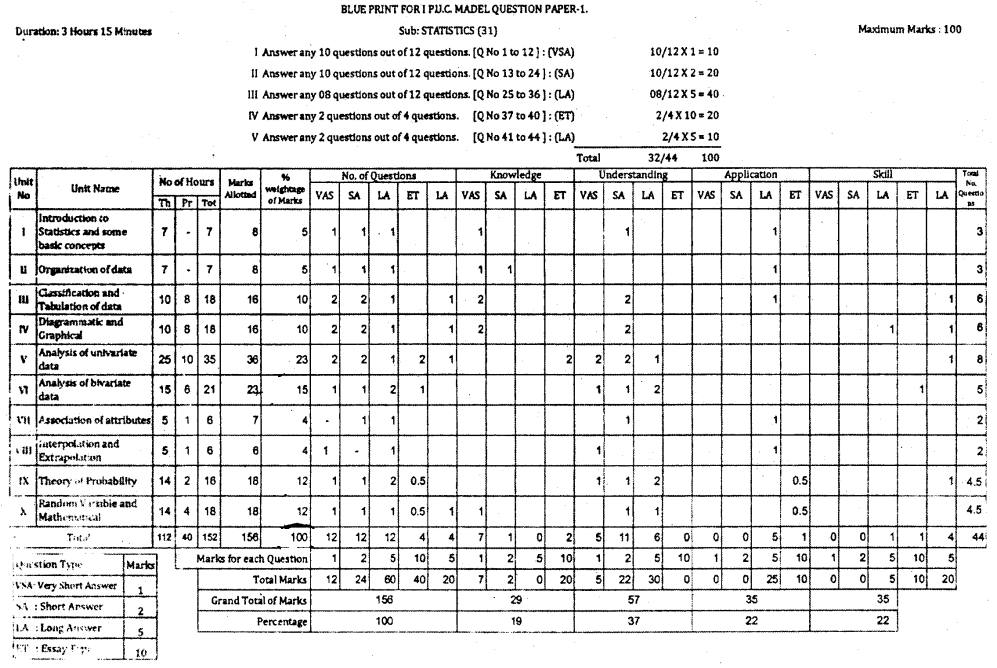
Karnataka 1st PUC Statistics Syllabus and Marking Scheme
Unit I Introduction to Statistics and Some Basic Concepts:
Meaning – Origin – Scope of Statistics. Definitions – in singular and plural sense. Characteristics, Branches, Functions and limitations of Statistics. Statistical applications in other subjects. Distrust of Statistics – causes and remedies. Some basic concepts – units, population, sample, qualitative characteristic, quantitative characteristic, attribute, variables (discrete and continuous), nominal scale and ordinal scale.
Unit II Organization of Data:
Statistical enquiry and its stages. Primary and Secondary data. Methods of collection of primary data, with merits and demerits. Essentials of a good questionnaire. Questionnaire and schedule with respect to their relative merits and demerits. Sources of secondary data. Census Enumeration and Sample Survey with respect to their relative merits and demerits. Pilot survey. Sampling – Methods of sampling – simple random sampling, stratified sampling and systematic sampling. Errors of sampling.
Unit III Classification and Tabulation:
Classification – Introduction, Meaning and objectives of classification, Types – (i) Chronological (Temporal) (ii) Geographical (Spatial) (iii) Qualitative (Simple and manifold) (iv) Quantitative, Explanation with examples.
Formation discrete and continuous frequency distributions: Explanation of range, class, class limits, class intervals inclusive, exclusive open-end classes, correction factors conversion of inclusive classes to exclusive classes, class mid-point, width of the class interval, formation of less than and more than cumulative frequency distributions, frequency density, relative frequency. Rules of classification. Formation of uni-variate and bi-variate frequency distributions.
Tabulation : Meaning, difference between classification and tabulation. Parts of a table- Format of a table and brief explanation of parts of table, Rules of tabulation, Types of tables-
(i) simple and complex (many-fold) tables (ii) General and special purpose tables; preparation of blank tables, tables with numerical information.
Unit IV Diagrammatic and Graphical Representation of Data:
Diagrams – Meaning, needs of diagrams, General rules of construction of diagrams – simple, multiple, component, percentage bars – problems and constructions. Component (sub divided) Pie diagram.
Graphs – Explanation, types – Histogram (Equal and Unequal width of class intervals), examples for obtaining mode from Histogram. Frequency Polygon and frequency curve – meaning, method of construction with and without histogram. Ogives – Meaning method of construction of both less than and more than Ogives. Examples. Obtaining the values of Median and Quartiles from less than Ogive. Comparison of tables and diagrams, difference between diagrams and graphs.
Unit V Analysis of Univariate Data:
(a) Measures of central tendency (Averages) – Meaning, objectives, definition of an average, definition of central tendency, essentials of a good average. Types of averages,
- Arithmetic mean – Definition, problems for both ungrouped and grouped data, one missing frequency problems, properties of mean with numerical applications, corrected mean in case of one wrong observation, merits and demerits.
- Median – Definition, problems for ungrouped and grouped data, one missing frequency problems, graphical solution of median, merits and demerits.
- Mode – Definition, problems for ungrouped and grouped data (excluding grouping method). Graphical solution of mode, merits and demerits, Empirical relationship among mean, median and mode.
- Geometric mean – Definition, problems for ungrouped and grouped data, problems on growth rates and interest rates, merits and demerits.
- Harmonic mean – Definition, problems for ungrouped and grouped data, problems on average speed, average number of days required for the completion of a given work, merits and demerits. Relationship between AM, GM and HM.
(b) Measures of position – Meaning, definitions of quartiles, deciles and percentiles. Problem on ungrouped and grouped data.
(c) Measures of dispersion – Meaning, objectives, definition, essentials of a good measure
of dispersion. Absolute and relative measures of dispersion.
Types – absolute and relative measures.
- Range – Definition, absolute and relative measures formulae, problems on ungrouped data.
- Quartile deviation – Definition, absolute and relative measures formulae, problems on ungrouped and grouped data.
- Mean deviation – Definition, absolute and relative measures formulae, problems on ungrouped and grouped data based on mean, median and mode.
- Standard deviation – Definition, problems on ungrouped and grouped data, properties of standard deviation with numerical applications and variance. Coefficient of variation for ungrouped and grouped data.
(d) Moments, Skewness and kurtosis-
- Moments – Meaning, definition of centrla moments, description of first four central moments. Formulae for β1, γ1, β2 and γ2.
- Skewness – Definition, types with diagram measures of skewness – Karl Pearson’s and Bowley’s coefficient of skewness for grouped data. Skewness based on moments (for given values of µ2 and µ3 finding β2).
- Kurtosis – Definition, explanation of kurtosis with neat diagram, measure of kurtosis based on moments (given the values of µ4 and µ2 finding β2).
Unit VI Analysis of Bivariate Data:
(a) Correlation – Definition, Types – Simple, multiple, partial. Causation – Spurious, positive, negative, perfect and no correlation explanation with examples. Significance of study of correlation analysis.
Measurement of correlation – scatter diagram explanation with charts, merits and demerits. Problems regarding construction of scatter diagram.
Karl Pearson’s coefficient of correlation – definition, formulae for ungrouped and grouped data. Properties of coefficient of correlation, interpretation. Problems – ungrouped and grouped data.
Spearman’s coefficient of rank correlation – without ties and tie (one or two), interpretation. Problems – with ties (One or two repeated ranks) and without ties.
(b) Regression – Definition, regression lines/equations of x and y and y on x. Properties of regression coefficient and regression lines/equations, Problems on ungrouped and grouped data, uses of regression analysis. Comparison between correlation and regression.
Unit VII Association and attributes:
Introduction, definition, notations – meaning and methods of association. Yule’s coefficient of association and its applications.
Unit VIII Interpolation and Extrapolation:
Meaning and utilities of interpolation and extrapolation. Binomial expansion method of interpolation (with one missing value) and Extrapolation for next successive value. Merits and demerits, applications.
Unit IX Theory of probability:
Introduction to probability, Definition of Experiment, Outcomes, Deterministic experiment, Random experiment, Sample space, Null event, Simple event, Compound event, Sure event with examples. Meaning of Favourable and Exhaustive outcomes, Equally likely events, Union and intersection of events, Mutually exclusive events, Complement of an event with examples. Meaning of Classical and Empirical/statistical methods of assigning probabilities. Classical/Mathematical, Empirical/Statistical and axiomatic definitions of probability. Statement and proofs (on basis of Mathematical definition) of P(Φ) = 0 P(S) = 1, 0 ≤ P(A) ≤ 1, P(A) + P(A’) = 1, Statement and proofs (on basis of Mathematical definition) of addition theorem of probability – for two non-mutually and mutually exclusive events. Definition and examples of independent, dependent events and conditional probability. Statement and proofs (on basis of Mathematical definition) of multiplication theorem of probability – for dependent and independent events and applications.
Unit X Random Variable and Mathematical expectation of a discrete random variable:
Definition with examples of discrete and continuous random variables. Definition of probability mass function and probability density function. Bivariate and marginal probability distributions definitions with examples.
Definition of Expected value/mean, variance and standard deviation of a discrete random variable. Related functions defined on a discrete random variable, applications (solving numerical and verbal problems), including the case of missing probabilities. Expectation and variance of following functions with proofs – a, aX, aX ± b where ‘a’ and ‘b’ are any two constants and related examples.
Statement and proofs of addition and multiplication theorem of Expectation. Covariance and correlation coefficient of bivariate random variables.
1st PUC Statistics List of Practicals
- Formation of Univariate and Bivariate Frequency Distributions
- Preparation of Blank Tables and Tables with Information
- Diagrammatic Representation of Data
- Graphical Representation of Frequency Distribution
- Measures of Central Tendency and Positions
- Measures of Dispersion
- Measures of Skewness
- Correlation
- Regression
- Association of Attributes, Interpolation and Extrapolation
- Probability Applications
- Mathematical Expectation
- Covariance and Correlation of Random Variables
We believe that the shared knowledge about Karnataka State Board solutions for 1st PUC Statistics Question Bank with Answers benefits you a lot in your exam preparation. If you need more information on the same then stay connected with our site & get updated details on KTBS Solutions PDF for all Subjects.
